Application of a mathematic model to evaluate the impact of temperature to plankton development processes in the pangasius (Pangasianodon hypophthalmus) production pond in Viet Nam

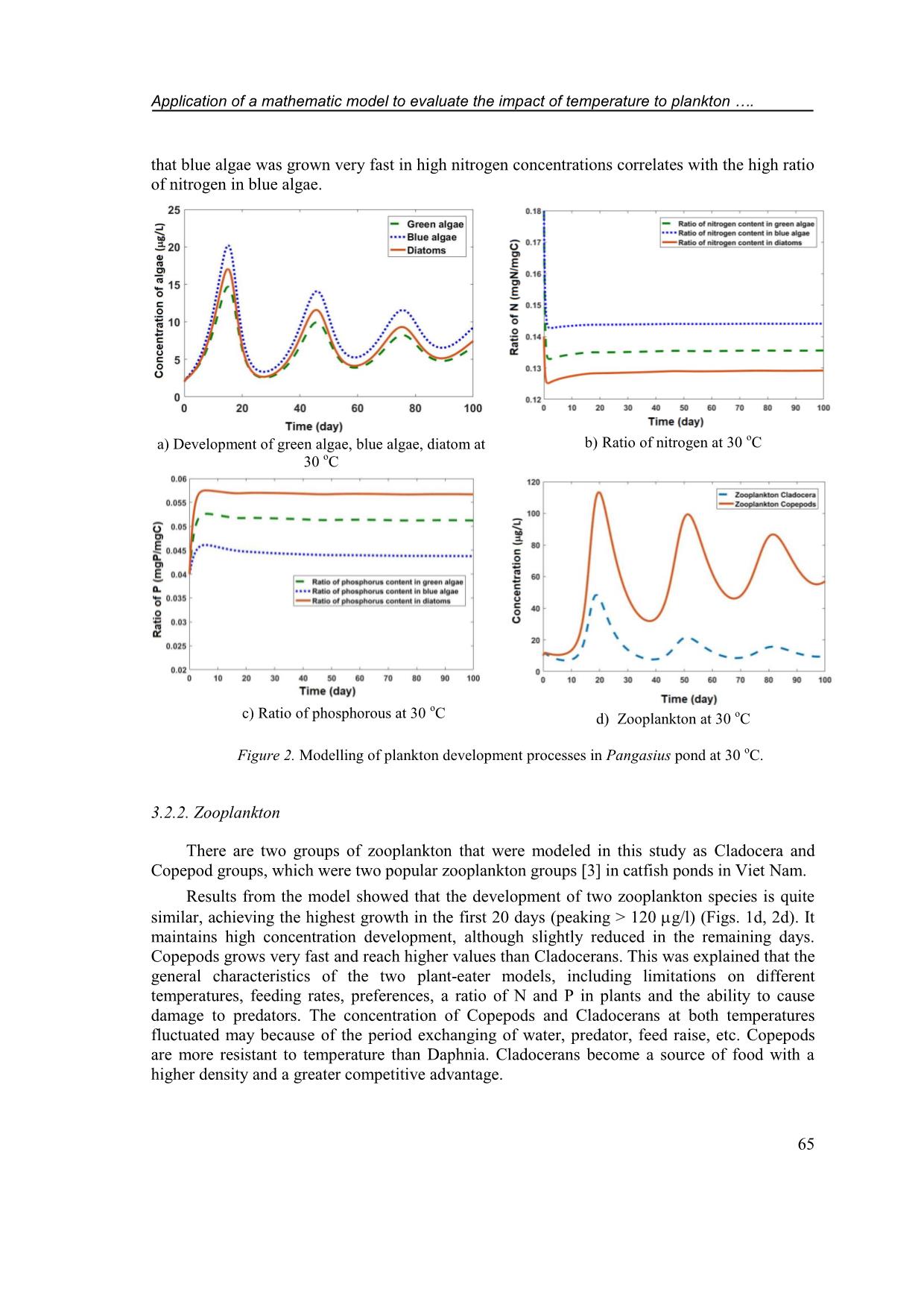
on samples were collected using a 57 m plankton net and 
fixed by formol-ether concentration 4 – 6 %. Samples were light shook and dropped 0.1 ml to 
glass for observing by microscope and classifying base on identification guide books [9]. 
The density of phytoplankon was determined by: 
 (1) 
in which: Y is the density of phytoplankton (individual/L); A: counting cell area; N: number of 
cells; T: Number of counted phytoplankton; VC: Concentrated volume (mL); VM: Volume of the 
sample (mL). 
The density of zooplankton was determined by: 
 (2) 
in which: P is the number of zooplankton (individual/m
3
); T: number of counted individual; A: 
area of the counted cell (mm
2
); N: number of cells; Vc: concentrated volume (m
3
); VM: volume 
of sample (m
3
). 
2.2. Data processing method 
Data was collected from the Pangasius pond in the field and previous study in Washington 
lake [10]. The mathematical model was used to simulate processes occurring in Pangasius 
ponds. Differential equations cannot be solved by conventional analytical methods but must be 
approximated by numerical methods as Runge-Kutta due to highly accurate, simple algorithm. 
The equations were solved numerically and then coded by Matlab 2018. The final step was used 
data sets and results from experiments or in previous studies to validate the model. 
Le Xuan Thinh, Dang Xuan Hien, Tran Van Nhan 
62 
The study on modeling at Washington lake presented a complex eutrophication model that 
has been developed to simulate plankton dynamics. This model is to be used for testing 
alternative managerial schemes, the inclusion of multiple elemental cycles (org. C, N, P, Si, O) 
and multiple functional phytoplankton (diatoms, green algae and cyanobacteria) and 
zooplankton (Copepods and Cladocerans) groups was deemed necessary [11]. 
2.3. Model assumption 
The object of the study is a close pond for intensive catfish farming in the Mekong Delta of 
Viet Nam, which has a simple physical structure that only represents the epilimnion pond. 
Nutritional resources for Pangasius fish was originated from autotrophic and heterotrophic food 
sources in ponds. The protein concentration of phytoplankton is assumed to be a constant. Water 
was exchanged 30 % every day from the sedimentation pond with assuming there is no loss of 
water for leakage, evaporation. There is no exchange of heat and light at the water surface in the 
pond. The survival rate of fish is constant, and catfish ponds are homogeneous block. 
2.4. Equation 
2.4.1. Phytoplankton 
The governing equation for algal biomass considers phytoplankton production and losses 
due to basal metabolism, settling and herbivorous zooplankton grazing. Nutrient, light and 
temperature impacts on phytoplankton growth are included using a multiplicative model. 
Phosphorus and nitrogen dynamics within the phytoplankton cells account for luxury uptake 
where phytoplankton nutrient uptake depends on both internal and external concentrations and is 
confined by upper and lower internal nutrient concentrations [11]. 
 ( ) 
 ∑ 
 (3) 
2.4.2. Zooplankton 
Based on the second trophic level (herbivory) of the model that is classified into two 
functional groups, which are labeled as “Copepods” and “Cladocerans”, and correspond to the 
general characteristics of a Diaptomus and Daphnia-like species, respectively. The general 
characteristics of the two herbivores modeled include different temperature limitations, feeding 
rates, food preferences, selectivity strategies, stoichiometries and vulnerability to predators. 
These differences drive their successional patterns and their interactions with the phytoplankton 
community. Copepods have a wider temperature tolerance than daphnids, which allows 
Copepods to dominate the winter zooplankton community and more promptly respond to the 
spring phytoplankton bloom [11]. 
 (∑ ) 
 ( ) 
 (4) 
Application of a mathematic model to evaluate the impact of temperature to plankton . 
63 
3. RESULTS AND DISCUSSION 
3.1. Matrix of mutual affecting factors in Pangasius ponds 
Based on the research of the relationship between C, P and N cycles; primary, secondary, 
tertiary production processes, the qualitative matrix was established for showing the relationship 
between the variables in the Pangasius ponds. 
The matrix of mutual affecting factors in the Pangasius farming pond is set as in Table 1. 
Table 1. The matrix of mutual affecting factors. 
 PHP ZOP DON MON PPA DOP MOP DOC MOC 
PHP 1 0 - - 1 1 - - - 
ZOP 1 1 1 1 1 
DON 1 1 1 1 
MON 1 1 1 
PPA 1/0 1 1 1 
DOP 1 1 1 1 
MOP 1 1 1 
DOC 1 1 1 1 
MOC 1 1 1 
Note: 1 – Impact; 0 – No impact. 
PHP: Phytoplankton; ZOP: Zooplankton; DON: Dissolved Organic Nitrogen; MON: Molecular 
Organic Nitrogen; PPA: Phosphate; DOP: Dissolved Organic Phosphate; MOP: Molecular Organic 
Phosphate; DOC: Dissolved Organic Carbon; MOC: Molecular Organic Carbon. 
3.2. Modeling of phytoplankton at different temperature 
Most of the mathematical equations and data sets in the model related to phytoplankton 
development were taken/inherited from Washington eutrophication lake and validated by the 
data set which was measured in the Vietnamese Pangasius ponds in accordance with the climate 
conditions in Viet Nam. The difference was that the Washington Lake model was built for 
thermal stratification lakes and surveyed in a wide temperature range, winters (5 - 10 degrees 
Celsius) and summers (15 - 20 degrees Celsius). In this study, the pond was always in the 
eutrophication state, without thermal stratification and at a narrower temperature range but with 
higher temperatures (25 - 35 
o
C). Therefore, the parameters of the plankton development 
processes in the Pangasius ponds were modeled at different temperatures (28, 30 
o
C). 
The results of modeling for phytoplankton and zooplanktons are the following: 
3.2.1. Phytoplankton 
Phytoplankton in ponds was divided into 3 groups, including: Green algae, Blue algae, 
Diatoms, because these are 3 common phytoplankton groups in Pangasius ponds in Viet Nam 
Le Xuan Thinh, Dang Xuan Hien, Tran Van Nhan 
64 
[3]; In addition, this division is based on the ability to convert N and P of each Phytoplankton 
group. Biomass Phytoplankton is attributed to Chlorophyll-a. 
The phytoplankton is simulated and corrected at two temperatures of 28 and 30 
o
C, for 
which the blue algae is the most grown algae (> 16 g/l), followed by green algae (> 13 g/l) 
and finally diatoms (> 11 g/l) (Fig 1a, 2a). All 3 types of algae reached the highest values from 
the 10th to the 20th day during the simulation period, which is also the time when the dissolved 
oxygen in the water increases. The amount of algae reaching such values correlates with the 
sunlight conditions that are assumed to be optimal for algae growth. Algae growing will create 
the food sources for zooplankton as well as Pangasius (catfish). 
a) Development of green algae, blue algae, diatom 
at 28 
o
C 
b) Ratio of nitrogen at 28 
o
C 
c) Ratio of phosphorous at 28 oC 
d) Zooplankton at 28 oC 
Figure 1. Modeling of plankton development processes in Pangasius pond at 28 
o
C. 
The growth restriction equation influenced by nutrients is a kinetic linear equation, which 
based on the dynamic that is the difference between the inorganic N (P) concentration in water 
and the existing N (P) concentration in algae. It also based on the maximum adsorption capacity 
of phytoplankton, the sub-variables which was the ratio of nitrogen and phosphorus in algae for 
quantification. The model results also showed the ratio of nitrogen and phosphorus in algae. 
With the ratio of nitrogen, blue algae have the biggest ratio (~ 0.141) then green algae (~ 0.132) 
and finally diatoms (~ 0.125) (Fig. 1b, 2b). During the production season, with the ratio of 
phosphorus, diatoms have the biggest ratio (~ 0.05) then green algae (~ 0.043) and finally blue 
algae (~ 0.005) (1c, 2c). The ratio also showed the different nutrition needs of algae, and it 
reflects algae growth in the existing available nutrient conditions in the pond. Namely, the fact 
Application of a mathematic model to evaluate the impact of temperature to plankton . 
65 
that blue algae was grown very fast in high nitrogen concentrations correlates with the high ratio 
of nitrogen in blue algae. 
a) Development of green algae, blue algae, diatom at 
30 
o
C 
b) Ratio of nitrogen at 30 oC 
c) Ratio of phosphorous at 30 
o
C d) Zooplankton at 30 oC 
Figure 2. Modelling of plankton development processes in Pangasius pond at 30 
o
C. 
3.2.2. Zooplankton 
There are two groups of zooplankton that were modeled in this study as Cladocera and 
Copepod groups, which were two popular zooplankton groups [3] in catfish ponds in Viet Nam. 
Results from the model showed that the development of two zooplankton species is quite 
similar, achieving the highest growth in the first 20 days (peaking > 120 g/l) (Figs. 1d, 2d). It 
maintains high concentration development, although slightly reduced in the remaining days. 
Copepods grows very fast and reach higher values than Cladocerans. This was explained that the 
general characteristics of the two plant-eater models, including limitations on different 
temperatures, feeding rates, preferences, a ratio of N and P in plants and the ability to cause 
damage to predators. The concentration of Copepods and Cladocerans at both temperatures 
fluctuated may because of the period exchanging of water, predator, feed raise, etc. Copepods 
are more resistant to temperature than Daphnia. Cladocerans become a source of food with a 
higher density and a greater competitive advantage. 
Le Xuan Thinh, Dang Xuan Hien, Tran Van Nhan 
66 
From the two investigated temperature points it is shown that both Copepods and 
Cladocerans were stronger developed in the lower temperature condition (28 
o
C), or in other 
words low temperature is close to the ideal development temperature for zooplankton. In which, 
Copepods are able to adapt to higher temperatures than Cladocerans, which is suitable for the 
initial assumptions for zooplankton. The state variables had the same development trend. The 
graphs were congruent with each other and differed in value at the peak when changing 
temperatures. 
It was also shown that at different temperatures 28 and 30 
o
C which was a suitable condition 
for Pangasius, and 28 
o
C is more suitable for the development of zooplankton and 
phytoplankton. The big change at two temperatures was observed at phytoplankton and 
zooplankton, meanwhile the little change was at nutrient components. 
4. CONCLUSIONS 
This study presents a model that simulates the plankton development processes in the 
Pangasius ponds. Based on the analysis and evaluation of influential matrices, the mathematical 
equations which describe the biological process development of fish in the pond (including 
zooplankton, phytoplankton) has been established. These equations are solved by the fourth-
order Runge-Kutta method and coded in Matlab programming language. 
The model allows to simulate plankton development processes and the effects of fish feed 
as well as to test the potential reaction of this pond to different water ambient temperatures. At 
28 and 30 
o
C, blue algae is the most grown algae (> 16 g/l) followed by green algae (> 13 g/l) 
and finally diatoms (> 11 g/l). All 3 types of algae reached the highest values from the 10th to 
the 20th day during the simulation period, which is also the time when the dissolved oxygen in 
the water increases. The model showed the ratio of nitrogen and phosphorus in algae, reflecting 
algae growth in the existing available nutrient conditions in the pond, such as blue algae was 
grown very fast in high nitrogen concentrations correlating with the high ratio of nitrogen in blue 
algae. The development of two zooplankton species achieved the highest growth in the first 20 
days (peaking > 120 g/l), Copepods grown very fast and reached higher values than 
Cladocerans. The results of this study may be beneficent to the production of catfish in the 
decision of temperature adjustment, feed component, time of feeding, etc. Although the result 
was good but it needs to have more study on the development of modeling with effects from 
external conditions of catfish pond in different seasons. 
Acknowledgements. The research funding from SWITCH-Asia Program (EU) for the project 
“Establishing a Sustainable Pangasius Supply Chain in Viet Nam” is acknowledged. 
REFERENCES 
1. Pham Dinh Don - Environmental pollution in aquaculture production and processing in 
Mekong Delta. Viet Nam Environment Administration Magazine No 6. 2014. (in 
Vietnamese); Online available at:  viewed on 
date 16th July 2019. 
2. Duong Nhut Long, Nguyen Anh Tuan, Le Son Trang - Intensive culture 
of Pangasius hypophthalmus in earthen ponds in the Mekong Delta, Viet Nam, Science 
Journal Can Tho University (2) (2004) 67-75 (in Vietnamese). 
Application of a mathematic model to evaluate the impact of temperature to plankton . 
67 
3. Phuong N. T., Ut V. N., Tung V. T., Hang N. T. T., Lien N. T. K., Oanh D. T. H., Huong 
D. T. T., and Morales E. J. - Water quality monitoring in striped catfish (Pangasianodom 
hypophthalmus) farms in the Mekong Delta, Viet Nam, College of Aquacultrue and 
Fisheries, Can Tho University, Viet Nam, Sustainable Fisheries Partnership, 2010. 
( _Water%20Quality2009_final.pdf), 29th 
May 2012, 1–19. 
4. Jingyang Z., Maryam R., Vincent C., George B. A. - Competition patterns among 
phytoplankton functional groups: How useful are the complex mathematical models, Acta 
Oecologica 33 (2008) 324-344. 
5. Jorgensen S. E. - Fundamentals of Ecological Modelling (2nd Edition), Elsevier, 
California (US) 1994. 
6. Jorgensen S. E. - Mathematical submodels in Water Quality Systems, Elsevier, California 
(US) (1989). 
7. Li L., Yakupitiyage A. - A Model for food nutrient dynamics of semi-intensive pond fish 
culture, Journal Aquaculture Engineering (Elsevier) 27 (2003) 9 - 38. 
8. APHA - Standard Methods for the Examination of Water and Wastewater. 19th Edition, 
American Public Health Association Inc., New York, 1995. 
9. Akihiko Shirota - The Plankton of South Viet Nam Fresh water and Marine Plankton 
(book) Published by Overseas Technical Cooperation Agency in Tokyo, Japan, 1966. 
10. Gurbir Perhar, George B. Arhonditsis, Michael T. Brett - Modeling zooplankton growth in 
Lake Washington: A mechanistic approach to physiology in a eutrophication model, 
Ecological Modelling 258 (2013) 101-121. 
11. George B. Arhonditsis, Michael T. Brett - Eutrophication model for Lake Washington 
(USA) Part I, Ecological Modelling 187 (2005) 140-178.