Decays of higgs in randall - Sundrum model

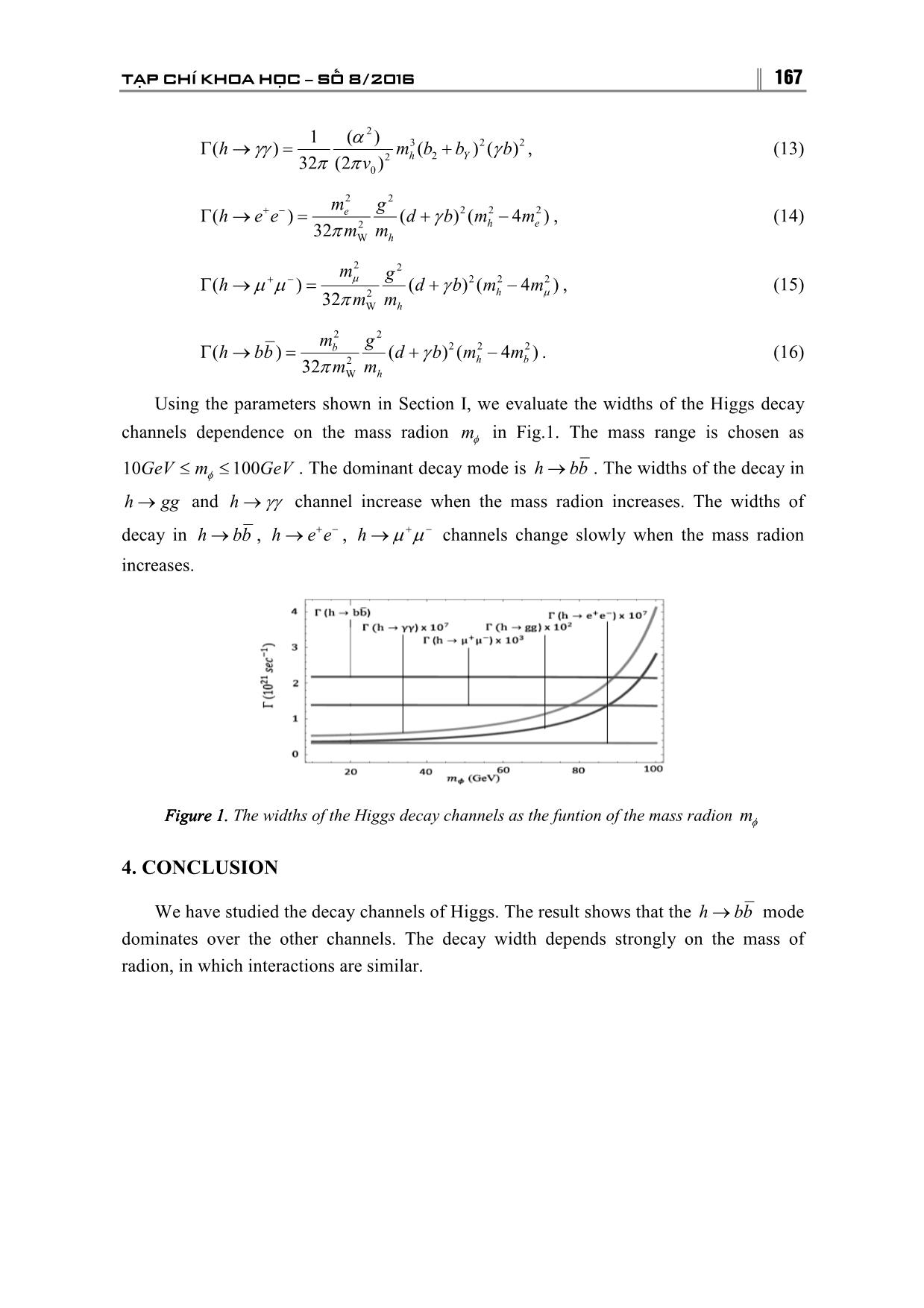
164 TRƯỜNG ĐẠI HỌC THỦ ĐÔ H NỘI 
 DECAYS OF HIGGS IN RANDALL  SUNDRUM MODEL 
 Dang Van Soa 1( 1), Dao Thi Le Thuy 2, Bui Thi Ha Giang 2 
 1Hanoi Metropolitan University 
 2Hanoi National University of Education 
 Abstract : In this paper, the decay widths of the Higgs under different channels in Randall  
 Sundrum model are studied in detail. The results showed that the decay width depends 
 strongly on the mass of radion. This suggests that the existence of radion in the Randall  
 Sundrum model is necessary. 
 KeywordsKeywords: Higgs boson, RandallSundrum model, decay width. 
1. INTRODUCTION 
 In 1999, Randall and Sundrum proposed a 5dimensional model for solving the gauge 
hierarchy problem [1,2]. The Randall – Sundrum (RS) model allows for a natural 
generation of Planckweak and fermion mass hierarchies [3]. Goldberger and Wise have 
proposed and attractive mechanism to stabilize the distance between two branes 
introducting a bulk scalar field which has scalar potentials on both branes [2]. In RS 
 1
model, the extra dimension is assumed to be located on a S/ Z 2 orbifold, which has two 
fixed points, φ = 0 and φ= π . They correspond to the high energy brane and the brane we 
 live on, respectively. Graviton is the only particle propagating through the bulk between 
 these two branes [4]. The spacetime metric ic given by: 
 ds2= e− 2ky η dx dx ν − dy 2 , (1) 
 ν
 where x ( = 0, 1, 2, 3) , y and k denote the coordinate of 4D spacetime, that of a fifth 
 dimension, and the AdS 5 curvature, respectively. The Minkowski metric is 
 −2ky
 ην =diag (1, −−− 1, 1, 1) and e is called a warp factor [1,2]. In four dimensional 
 effective theory of RS model, there are two new particles beyond the Standard model. One 
(1) Nhn bài ngày 8.8.2016; gi phn bin và duyt ñăng ngày 15.9.2016 
 Liên h tác gi: Đng Văn Soa; Email: dvsoa@daihocthudo.edu.vn 
TẠP CHÍ KHOA HỌC −−− SỐ 8/2016 165 
is a spin2 graviton and a scalarfield radion φ which is metric fluctuation along the extra 
 dimension. 
 Having determined the vacuum structure of the model, we discuss the possibility of 
mixing between gravity and electroweak sector. The gravityscalar mixing is described by 
the following action [5, 6, 7] 
 S= −ξ dx4 − gRg( ) HHˆ+ ˆ , (2) 
 ξ ∫ vis vis
 Where R( g vis ) is the Ricci scalar for the metric induced on the visible brane, 
 ν2 ν ν ˆ
 gvis=  b ()( xη + ε h ) . H is the Higgs filed in the 5D context before rescaling to 
 canonical normalization on the brane. The parameter ξ denotes the size of the mixing term 
[110]. With ξ ≠ 0, neither a pure Higgs boson not pure radion mass eigenstate. 
 We difine the mixing angle θ by: 
 2
 mh
 θ= γξ Z 0
 tan 2 12 2 22 22 . (3) 
 mφ − mh ( Z − 36ξ γ )
 0 0
 Where: 
 Z2≡+16ξγ 2 (16) −≡− ξ β 36 ξγγ 22 , =Λ v / . (4) 
 0 φ
 In terms of these quantities, the new fields h and φ are the states that diagonalize the 
kinetic energy and have canonical normalization with: 
 6ξγ 6 ξγ
 h0 =−(cosθ sin θ ) h ++ (sin θ cos θφ ) ≡+ dhc φ , (5) 
 Z Z
 φ h
 φ0 =−cos θ + sin θ ≡+ a φ bh . (6) 
 Z Z
 The corresponding masssquared eigenvalues are [11] 
 21 2 2 2 22222
 mhφφ= m +±+β m h[ m φ β m h ] − 4 Zmm φ h . (7) 
 , 2 ( 0 0 0 0 00 )
 2Z
 When ξ ≠ 0, there are four independent parameters that must be specified to fix the 
 state mixing parameters a, b, c, d of Eqs. (5) and (6) defining the mass eigenstates 
 Λ ,m , m , ξ . (8) 
 φh φ
 m0
 We consider the case of Λφ = 5TeV and = 0.1 , which makes the radion 
 M P
 stabilization model most natural [12]. 
166 TRƯỜNG ĐẠI HỌC THỦ ĐÔ H NỘI 
 The search experiments of the Higgs boson at the LHC give stringent constraints on 
the parameters of the radion (a radion mass mφ and a scale parameter Λφ ). The recently 
discovered 125 GeV scalar at the LHC RunI [13, 14], behaves like the SM Higgs boson 
and this fixes the last free parameter of the SM Lagrangian [15]. 
 In this paper, we study the decay channels of Higgs. This paper is organised as 
follows. In Sec.II, we briefly review the interactions of Higgs to SM fields. In Sec.III, the 
widths of the Higgs decay channels and our numerical results are shown. Sec.IV is devoted 
to summary and discussion. 
2. I NTERACTIONS 
 n
 We turn to the important interactions of the h, φ and hν . We begin with the gg 
couplings of the h and φ . The h0 has standard gg or fermionic coupling and the φ0 has 
 φ0 
 ZZ or fermionic coupling from interaction − T using the Yukawa interaction 
 Λφ
 
 contributions of T . The results are obtained by: 
 ab ν ν 
 gggh= C g δ[( kk12 ) η − kk 12 ] , (9) 
 g= Ckk[( )η ν − kk ν  ] , (10) 
 γγh γ 12 12
 g me
 geeh = −( d + γ b ) , (11) 
 2 mW
 where g and cW denote the SU(2) gauge coupling and cosine of the Weinberg angle, 
 respectively. There, 
 αs
 Cg =−[( dbF +γ )∑ 1/2 ( τi ) − 2 ba 3 γ ], 
 4πv i
 αs 2 i
 Cγ =−[( dbeNF +γ )∑ i c1 ()( τ i −+ bbYa 2 )] γ 
 2πv i 
3. D ECAY OF HIGGS 
 We calculate the decay widths of the Higgs to the SM particles as follows: 
 1 (−α 2 )
 Γ→=(hgg )s mbb3 (2) − γ 2 , (12) 
 32π (4 π v ) 2 h 3
 0
TẠP CHÍ KHOA HỌC −−− SỐ 8/2016 167 
 1 (α 2 )
 Γ→=(hγγ ) mbbb3 ()() + 2 γ 2 , (13) 
 32π (2 π v ) 2 h2 Y
 0
 m2 g 2
 Γ→=(hee+ − )e ()(4) dbmm +γ 2 2 − 2 , (14) 
 32 π m2 m h e
 W h
 m2 g 2
 Γ→=(h+  − ) ()(4) dbmm + γ 2 2 − 2 , (15) 
 32 π m2 m h 
 W h
 m2 g 2
 Γ→=(hbb )b ( dbmm +γ )(4)2 2 − 2 . (16) 
 32 π m2 m h b
 W h
 Using the parameters shown in Section I, we evaluate the widths of the Higgs decay 
channels dependence on the mass radion mφ in Fig.1. The mass range is chosen as 
 10GeV≤ mφ ≤ 100 GeV . The dominant decay mode is h→ bb . The widths of the decay in 
 h→ gg and h → γγ channel increase when the mass radion increases. The widths of 
decay in h→ bb , h→ e+ e − , h → +  − channels change slowly when the mass radion 
increases. 
 FigurFigureeee 11..1. The widths of the Higgs decay channels as the funtion of the mass radion mφ 
4. CONCLUSION 
 We have studied the decay channels of Higgs. The result shows that the h→ bb mode 
 dominates over the other channels. The decay width depends strongly on the mass of 
 radion, in which interactions are similar. 
168 TRƯỜNG ĐẠI HỌC THỦ ĐÔ H NỘI 
 REFERENCES 
1. L. Randall and R. Sundrum (1999), Phys. Rev. Lett. 83, 3370, arxiv: hepph/9905221. 
 2. L. Randall and R. Sundrum (1999), Phys. Rev. Lett . 83, 4690, arxiv: hepph/9906064. 
 3. W. D. Goldberger and M. B. Wise (1999), Phys. Rev. Lett. 83, 4962, arxiv: hepph/9907447. 
 4. S. A. Li, C. S. Li, H. T. Li and J. Gao (2015), "Constraints on RandallSundrum model from 
 the events of dijet production with QCD nexttoleading order accuracy at the LHC", 
 [arXiv:1408.2762v2 [hepph]]. 
 5. J.J. Van der Bij (1994), Acta Phys. Podon, B 25, 827. 
 6. R. Raczka, M. Pawlowski (1994), Found. Phys. 24, 1305. 
 7. G. F. Giudice, R. Rattazzi and J. D. Wells (2001), "Graviscalars from higher dimensional 
 metrics and curvature Higgs mixing", Nucl. Phys . B 595, 250 [hepph/0002178]. 
 8. D. V. Soa, D. T. L. Thuy, N. H. Thao and T. D. Tham (2012), Mod. Phys. Lett. A, Vol.27, 
 N0.2, 1250126. 
9. M. Chaichain, A. Datta, K Huitu and Z. Yu (2002), Phys. Lett . B 524, 161. 
10. K. Cheung, C. S. Kim and J. h. Song, (2003), "A Probe of the radion Higgs mixing in the 
 RandallSundrum model at e+ e colliders," Phys. Rev. D 67, 075017, [hepph/0301002]. 
11. T. Han, G. D. Kribs and B. McElrath, (2001), Phys. Rev . D 63, 076003. 
12. H. Davoudiasl, J. L Hewett and T. G. Rizzo (2001), Phys. Rev. D 63, 075004. 
13. ATLAS Colllaboration, G. Aad et al (2012), Phys. Lett, B 716, 129, arxiv: hepph/1207.7214. 
14. CMS Colllaboration, S. Chatrchyan et. Al (2012), Phys. Lett. B 716, 3031, arxiv: hep
 ph/1207.7235. 
15. Goutam Das, Prakash Mathews (2015), Phys. Rev. D 92, 094034. 
 QUÁ TRÌNH PHÂN RÃ HIGGS TRONG MÔ HÌNH 
 RANDALL  SUNDRUM 
 Tóm tttttt: Trong bài báo này, chúng tôi nghiên cu chi tit quá trình rã Higgs boson thành 
 các cp gg,,γγ e+− e ,  +  − , bb . C th, chúng tôi ñã tính ñưc biu thc gii tích ca 
 ñ rng phân rã và sau ñó kho sát s ph thuc ñ rng phân rã theo khi lưng ca 
 radion. Kt qu thu ñưc cho thy, ñi vi quá trình rã Higgs thành gg , γγ thì ñ rng 
 phân rã tăng khi khi lưng radion tăng. Còn ñi vi quá trình rã Higgs thành 
 e+− e, +  − , bb thì ñ rông thay ñi không ñáng k khi khi lưng radion thay ñi. Đ 
 rng phân rã thu ñưc là ln nht ñi vi quá trình rã h→ bb . 
 TTTT khoákhoá: Higgs boson, mô hình RandallSundrum, ñ rng phân rã.