Performance analysis of rf energy harvesting cooperative communication networks with DF scheme
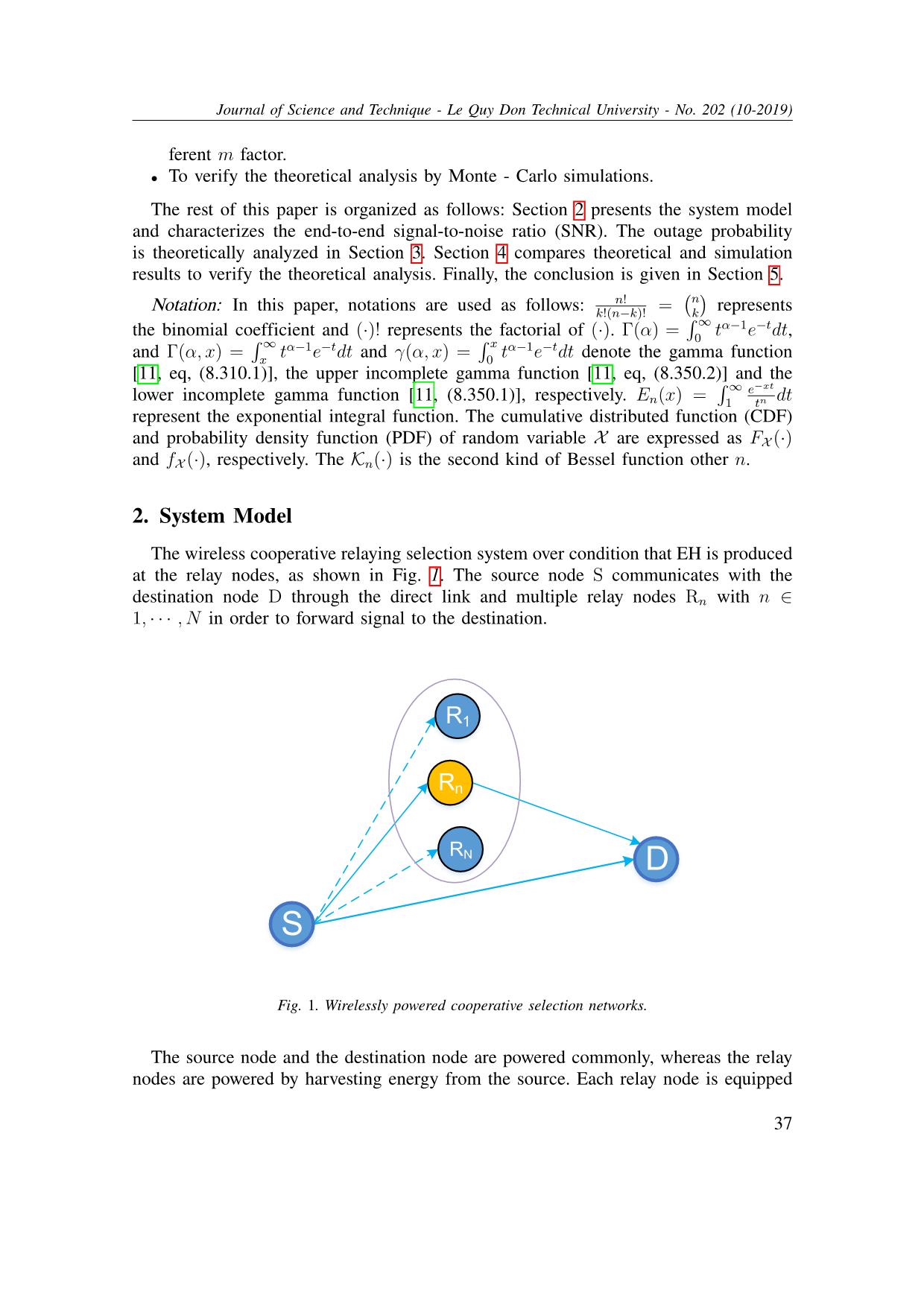
Wireless energy transfer cooperative communication systems are analyzed in this paper.
In these systems, a source node can communicate with a destination node directly or via the
selected relay nodes, while relay nodes harvest energy from radio frequency for forwarding the
received signal. In addition, the decode and forward (DF) protocol is applied to relay nodes,
and selection combination technique is employed at the destination in order to select the
best relay node. The system performance is presented by outage probability expressions over
independent and identically distributed (i.i.d) Nakagami-m channel model. The theoretical
analysis and the closed-form expression of outage probability are derived and compared with
Monte-Carlo simulations. The simulation results are similar to the theoretical analysis results,
it verifies our proposed derivation method.

Trang 1

Trang 2
Trang 3

Trang 4

Trang 5

Trang 6

Trang 7
Trang 8

Trang 9
Trang 10
Tải về để xem bản đầy đủ
Tóm tắt nội dung tài liệu: Performance analysis of rf energy harvesting cooperative communication networks with DF scheme

2 Km1+k−t 2 . (21)
λ2m1 (n + 1) λ1λ2
n(m −1)
N−1 1 m2−1 m1+k t n n
X X X 1 N − 1 m1 m2φ (−1) bk N
I(a, φ) = 1− N−1
t! n λ1 λ2
n=0 k=0 t=0 Γ(m1)
∞ ` `
X (−1) m2φ
× Ψ(x). (22)
`! λ2
`=0
where
m1−k+t+`
m1 (1+n) m1 (1+n) γth
Γ m1 +k−t−`, ,
λ1 λ1PS
with m1 +k−t−`>1, (23a)
m (1+n)γ
q+1 q 1 th
exp − λ P
(−1) m1 (1 + n) m1 (1 + n) γth 1 S gX
Ψ(x)= Ei − + q ,
q! λ1 λ1PS γth
P
S
j j
j m1(1+n) γ
q−1 (−1) th
X X λ1 PS
with g = , q = m + k − t − ` < 1. (23b)
q (q − 1) ··· (q − j) 1
j=0
Proof: We rewrite (13) to become an independent product of two probability com-
2 2 2
ponents, and let X = max |h1,i| , Y = |hRnD| and Z = |hSD| be the random
i=1,...,N
variables Gamma distribution, which are modeled as X ∼ G (m1, β1), Y ∼ G (m2, β2)
and Z ∼ G (m0, β0), respectively.
Substituting (17) and (2) into (20), we get I (a, φ) that is showed by the equations
(24a).
n(m −1)
1 m2−1 N−1 m1+k t n n
X X 1 X N − 1 m1 φm2 N(−1) bk
I(a, φ) = 1− N−1 J(x)
t! n λ1 λ2 Γ(m1)
k=0 t=0 n=0
(24a)
∞
Z
m +k−t−1 φm2 m1(n + 1)x
where J(x) = x 1 exp − − dx. (24b)
xλ2 λ1
a
42
Journal of Science and Technique - Le Quy Don Technical University - No. 202 (10-2019)
In the case of high transmit power, i.e a = γthN0 → 0, by applying [11, 3.471.9],
PS→∞
we obtain I (a, φ) as in (21), and then by replacing back (21) into (20), we obtain the
approximation of outage probability expression as in (24b). However, this approximation
is unsuitable for low transmit power which is basically applied for EH system. To obtain
the closed-form of the outage probability expression for general case of transmit power,
∞
� a P (−1)t � a t
the exponential function is expanded by Taylor algorithm: exp − x = t! x .
t=0
Therefore, the J (x) in (24b) is rewritten as
∞
∞ ` ` Z
X (−1) m2φ m +k−t−`−1 m1 (1 + n) x
J (x) = x 1 exp − dx . (25)
`! λ2 λ1
`=0 a
| {z }
Ψ(x)
Finally, by applying [11, Eq:3.351.211, Eq:3.351.4] for the integral term in (25), we
have the Ψ(x) that is given in (23a) and (23b). The proof of Proposition is completed.
4. Simulation Results
In this section, we show the Monte - Carlo simulation results and compare them with
our theoretical analysis. We assume that the R is fixed as 1 bit/s/Hz, η = 1 (perfect
current converter), PS is constant, and α = 0.3. The distance from S to D is normalized
to unit value. We also assume that the relay node cluster is at the middle of the source
and the destination. Moreover, all channels are identical independent distribution (i.i.d).
For the sake of simplicity, the average channel gains are set as λ1i = λ2j = λ0 = 1.
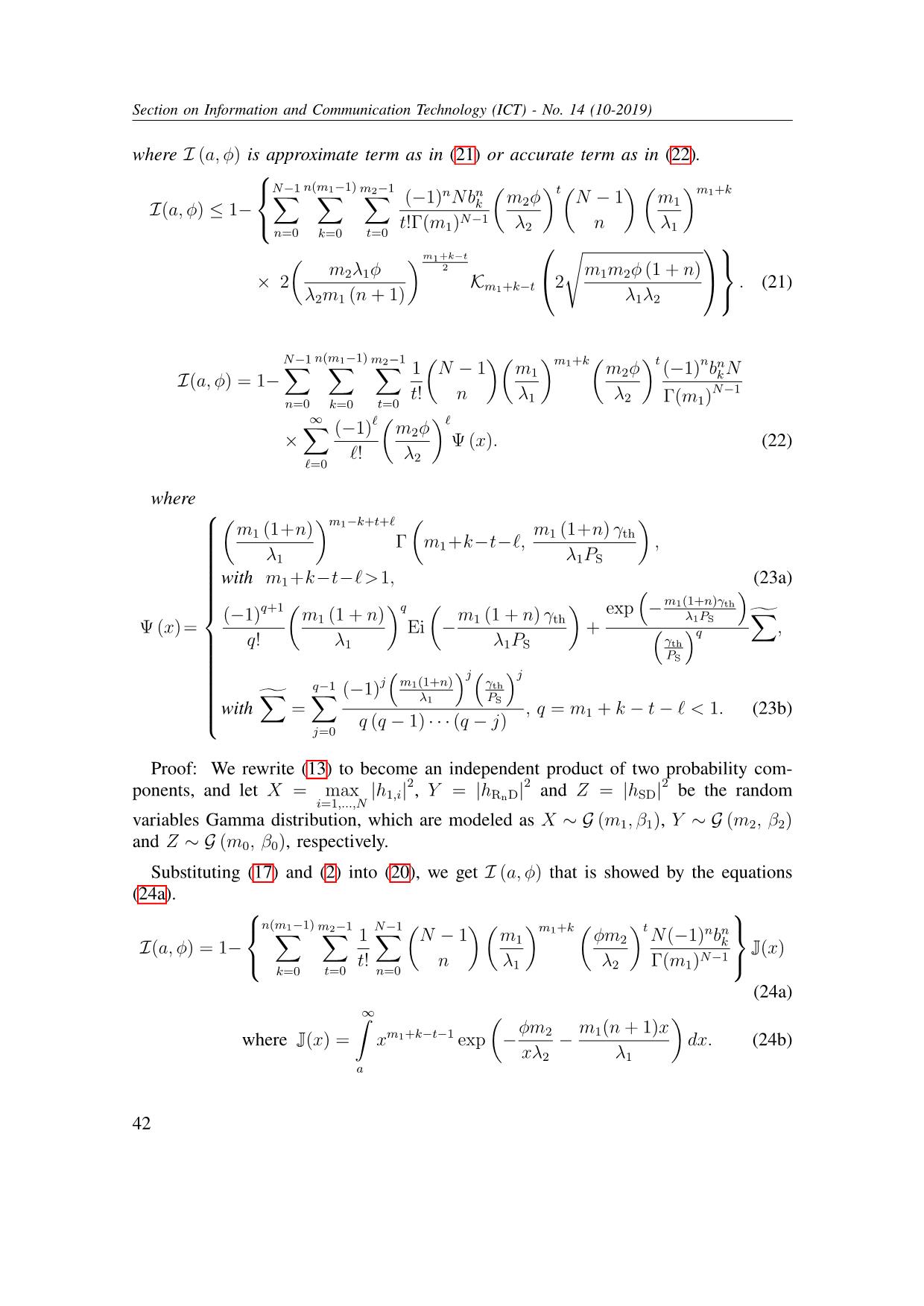
Fig. 3 illustrates the outage probability versus the average transmit power of the S.
In order to reduce complexity, we choose [m1 m2 m3] = [2 2 2], where m1, m2, m3 are
the distribution parameters of the links S-R, R-D and S-D, respectively. The number
of relays is changed within [1,5]. The result in Fig. 3 shows that the channel gain
is increased by increasing the number of relays. The reason is, the selected best relay
provides the best channel from the source to the relay in order to achieve better decoding
performance as well as higher RF EH from the source in the first phase. Furthermore,
it is clear that the theoretical analysis is perfect match with the simulation, it confirms
the correctness of the proposed analysis approach.
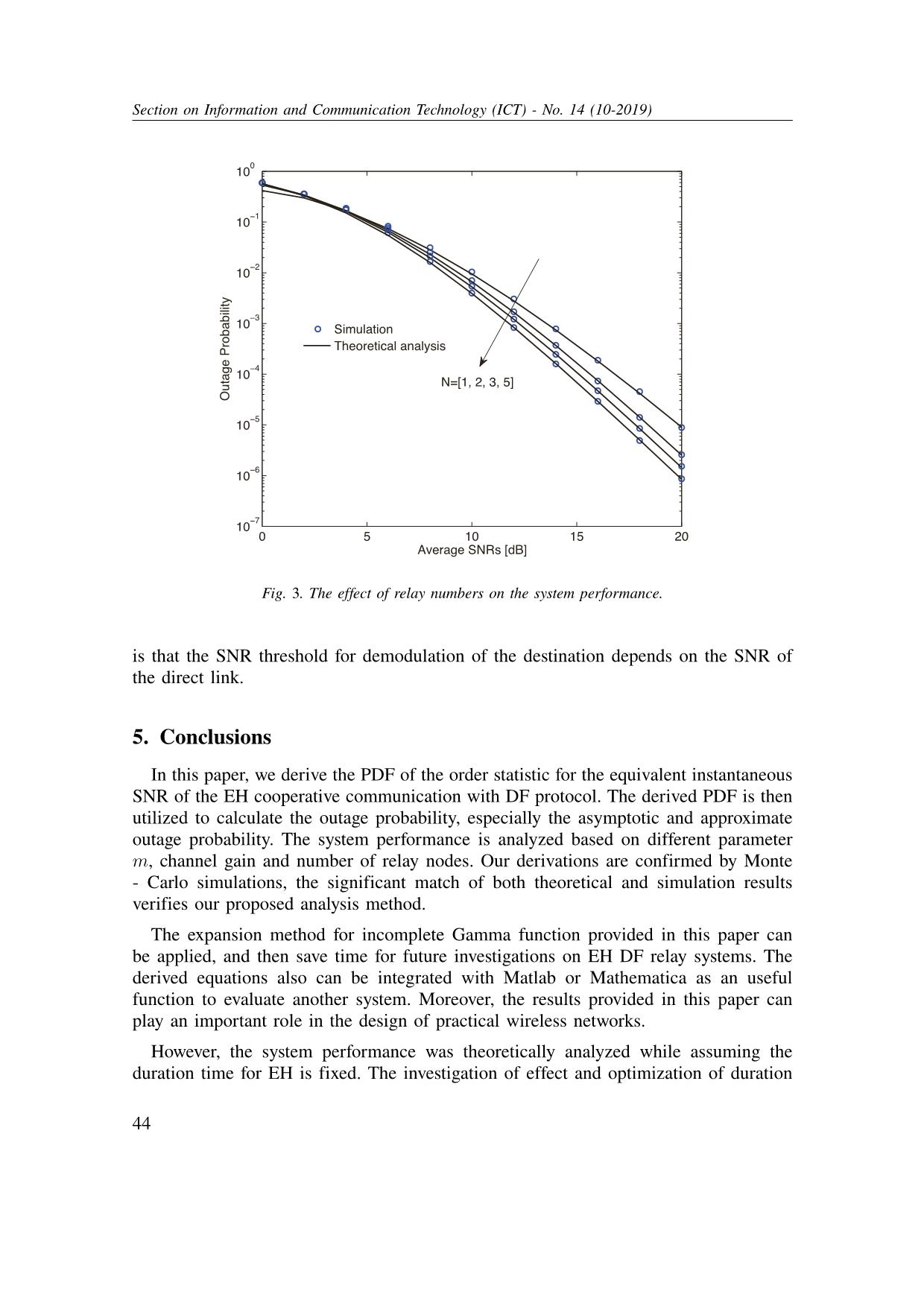
Fig. 4 demonstrates the outage probability of the EH cooperative communication
system with respect to the different parameters m, while other parameters are set to
be the same as in Fig. 3. In this figure, the excellent agreement between the analytical
result and simulation result is also observed. When the parameter m is increased, the
outage probability decreases. It is explained that the other diversity is improved by
the d = min(mx , my), and then the system performance is improved significantly. In
addition, when the quality of direct link is better than that of the forward link, the
parameter m of forward link unaffects the system performance. The reason of this state
43
Section on Information and Communication Technology (ICT) - No. 14 (10-2019)
0
10
−1
10
−2
10
−3
10 Simulation
Theoretical analysis
−4
10
N=[1, 2, 3, 5]
OutageProbability
−5
10
−6
10
−7
10
0 5 10 15 20
Average SNRs [dB]
Fig. 3. The effect of relay numbers on the system performance.
is that the SNR threshold for demodulation of the destination depends on the SNR of
the direct link.
5. Conclusions
In this paper, we derive the PDF of the order statistic for the equivalent instantaneous
SNR of the EH cooperative communication with DF protocol. The derived PDF is then
utilized to calculate the outage probability, especially the asymptotic and approximate
outage probability. The system performance is analyzed based on different parameter
m, channel gain and number of relay nodes. Our derivations are confirmed by Monte
- Carlo simulations, the significant match of both theoretical and simulation results
verifies our proposed analysis method.
The expansion method for incomplete Gamma function provided in this paper can
be applied, and then save time for future investigations on EH DF relay systems. The
derived equations also can be integrated with Matlab or Mathematica as an useful
function to evaluate another system. Moreover, the results provided in this paper can
play an important role in the design of practical wireless networks.
However, the system performance was theoretically analyzed while assuming the
duration time for EH is fixed. The investigation of effect and optimization of duration
44
Journal of Science and Technique - Le Quy Don Technical University - No. 202 (10-2019)
0
10
[mm m ] = [2 2 1]
SR RD SD
−1
10
[mm m ] = [2 1 2]
SR RD SD
−2
10
−3
10
Simulation
Outage Probability Theoretical analysi
−4 s
10
−5
10
m m =[2 2 2]
SR RD SD
−6
10
0 5 10 15 20
Average SNRs [dB]
Fig. 4. The outage probability with different values of parameter m.
time for EH is left for the future work.
Acknowledgment
This research is funded by Vietnam National Foundation for Science and Technology
Development (NAFOSTED) under Grant No. 102.04-2017.311
References
[1] X. Lu, P. Wang, D. Niyato, D. I. Kim, and Z. Han, “Wireless networks with RF energy harvesting: A
contemporary survey,” IEEE Commun. Tutorials, vol. 17, no. 2, pp. 757–789, Mach. 2015.
[2] L. Zhao, X. Wang, and K. Zheng, “Downlink hybrid information and energy transfer with massive MIMO,”
IEEE Commun. Mag, vol. 15, no. 2, pp. 1390–1322, Feb. 2016.
[3] R. Vahidnia, A. Anpalagan, and J. Mirzaei, “Diversity combining in bi-directional relay networks with energy
harvesting nodes,” IET Commun, vol. 10, no. 2, pp. 207–211, Feb. 2016.
[4] N. T. Do, V. N. Q. Bao, and B. An, “A relay selection protocol for wireless energy harvesting relay networks,”
Proc. 2015 12th Adv Tech Commun (ATC), vol. 10, no. 2, pp. 243–247, Oct. 2015.
[5] P. N. Son, H. Y. Kong, and A. Anpalagan, “Exact outage analysis of a decode-and-forward cooperative
communication network with Nth best energy harvesting relay selection,” Annals of Telecommunications, vol. 71,
no. 5-6, pp. 251–263, June 2016.
[6] Y. Chen, “Energy-harvesting af relaying in the presence of interference and Nakagami-fading,” IEEE Trans.
Commun., vol. 15, no. 2, pp. 1008–1017, Feb. 2016.
45
Section on Information and Communication Technology (ICT) - No. 14 (10-2019)
[7] Y. Dong, M. Hossain, and J. Cheng, “Performance of wireless powered amplify and forward relaying over
Nakagami-m fading channels with nonlinear energy harvester,” IEEE Commun. Lett., vol. 20, no. 4, pp. 672 –
675, Apr. 2016.
[8] T. M. Hoang, T. T. Duy, and V. N. Q. Bao, “On the performance of non-linear wirelessly powered partial
relay selection networks over rayleigh fading channels,” Proc. 2016 3rd Nat Found Science and Tech Dev Conf
(NICS), vol. 10, no. 2, pp. 6–11, Sept. 2016.
[9] T. M. Hoang, N. T. Tan, N. H. Hoang, and P. T. Hiep, “Performance analysis of decode-and-
forward partial relay selection in noma systems with rf energy harvesting,” Wireless Networks, pp. 1–11,
https://doi.org/10.1007/s11 276–018–1746–8, May 2018.
[10] T. M. Hoang, V. V. Son, N. C. Dinh, and P. T. Hiep, “Optimizing duration of energy harvesting for downlink
noma full-duplex over nakagami-m fading channel,” Int. J. Electron. Commun. (AEU)¨ , vol. 95, pp. 199–206,
Oct. 2018.
[11] D. Zwillinger, Table of integrals, series, and products. Elsevier, 2014.
[12] V. N. Q. Bao and H. Y. Kong, “Performance analysis of decode-and-forward relaying with partial relay selection
for multihop transmission over Rayleigh fading channels,” J. Commun. Netw., vol. 12, no. 5, pp. 433–441, Oct.
2010.
[13] A. A. Nasir, X. Zhou, S. Durrani, and R. A. Kennedy, “Relaying protocols for wireless energy harvesting and
information processing,” IEEE Trans. Wireless Commun., vol. 12, no. 7, pp. 3622–3636, July. 2013.
[14] T. Q. Duong, V. N. Q. Bao, and H.-J. Zepernick, “On the performance of selection decode-and-forward relay
networks over Nakagami-m fading channels,” IEEE Commun. Lett., vol. 13, no. 3, pp. 172–174, Mar. 2009.
[15] D. Senaratne and C. Tellambura, “Unified exact performance analysis of two-hop amplify-and-forward relaying
in Nakagami m fading,” IEEE Trans. Veh. Technol., vol. 59, no. 3, pp. 1529–1534, Dec. 2010.
[16] I. Krikidis, S. Timotheou, and S. Sasaki, “RF energy transfer for cooperative networks: Data relaying or energy
harvesting?” IEEE Commun. Lett., vol. 16, no. 11, pp. 1772–1775, Nov. 2012.
[17] S. Sudevalayam and P. Kulkarni, “Energy harvesting sensor nodes: Survey and implications,” IEEE Commun.
Surveys Tuts., vol. 13, no. 3, pp. 443–461, July. 2011.
[18] I. Krikidis, G. Zheng, and B. Ottersten, “Harvest-use cooperative networks with half/full-duplex relaying,” in
Wirel Commun and Netw Conf (WCNC). IEEE, 7-10 Apr. 2013, pp. 4256–4260.
[19] T. T. Duy and H.-Y. Kong, “Performance analysis of incremental amplify-and-forward relaying protocols with
nth best partial relay selection under interference constraint,” Wireless Personal Communications, vol. 71, no. 4,
pp. 2741–2757, 2013.
[20] T. T. Duy, T. Q. Duong, D. B. da Costa, V. N. Q. Bao, and M. Elkashlan, “Proactive relay selection with joint
impact of hardware impairment and co-channel interference,” IEEE Transactions on Communications, vol. 63,
no. 5, pp. 1594–1606, 2015.
[21] T. T. Duy and H.-Y. Kong, “Performance analysis of hybrid decode-amplify-forward incremental relaying
cooperative diversity protocol using SNR-based relay selection,” IEEE Commun. Lett., vol. 14, no. 6, pp.
703–709, Jan. 2012.
[22] G. Fedele, “N-branch diversity reception of mary DPSK signals in slow and non selective Nakagami m fading,”
IEEE Trans. Commun. Technol., vol. 7, no. 2, pp. 119–123, Mar. 1996.
Manuscript received 19-9-2019; Accepted 18-12-2019.
Hoang Duc Vinh received B.E degree in Electronics and Communications Engineering from
University of Transport and Communications in 2004, M.E degree in Radio-Electronics En-
gineering of Le Quy Don Technical University in 2011. He had worked at Department of
Information and Communications of Nghe An province from 2005 to 2011, and at Ministry of
Information and Communications of Viet Nam from 2011 to 2016. Now he is a Ph.D candidate
of Le Quy Don Technical University, Hanoi, Vietnam. His reseach interests lie in the area of
physical layer and spectrum management of wireless systems..
46
Journal of Science and Technique - Le Quy Don Technical University - No. 202 (10-2019)
Vu Van Son received the B.E.E. degree from the Le Quy Don Technical University, in
2004; received the Ph.D. degree in Radio-physics, Electronic and Medicine Engineering from
the Vladimir State University, Russia, in 2009. His previous research interests have included
stochastic processes, wireless communications, pattern recognition, and neural networks. His
current work is mainly focused on wireless communications, with special emphasis on model-
ing, estimation, and efficient simulation of wireless channels, and system performance analysis.
Bui Anh Duc received the B.S. in 2013 in Telecommunication University and M.S from Le
Quy Don University, Ha Noi, Vietnam in 2017. His research interests include wireless body
area network cooperative communication.
Tran Manh Hoang received the B.S. degree in Communication Command from Telecom-
munications University, Ministry of Defense, Vietnam, in 2002, and the B. Eng. degree in
Electrical Engineering from Le Quy Don Technical University, Ha Noi, Vietnam, in 2006. He
obtained the M.Eng. degree in Electronics Engineering from Posts and Telecommunications,
Institute of Technology, (VNPT), Vietnam, in 2013. He is currently pursuing the Ph.D degree
at Le Quy Don Technical University, Hanoi, Vietnam. His research interests include energy
harvesting, Non-orthogonal Multiple Access, and signal processing for wireless cooperative
communications.
47
Section on Information and Communication Technology (ICT) - No. 14 (10-2019)
Pham Thanh Hiep received the B.E degree in Communications Engineering from National
Defence Academy, Japan, in 2005; received the M.E and Ph.D degree in Physics, Electrical
and Computer Engineering from Yokohama National University, Japan, in 2009 and 2012,
respectively. He was an associate researcher at Center for Future Medical Social Infrastructure
Based on Information Communications (MICT cener) of Yokohama National University during
2012-2015. He is currently working as lecturer at Le Quy Don Technical University, Ha Noi,
Viet Nam. His reseach interests lie in the area of wireless information and communications
technologies.
PHÂN TÍCH HIỆU NĂNG HỆ THỐNG HỢP TÁC
GIẢI MÃ CHUYỂN TIẾP ỨNG DỤNG THU THẬP
NĂNG LƯỢNG VÔ TUYẾN
Tóm tắt
Bài báo phân tích hoạt động truyền tải thông tin và năng lượng đồng thời cho mạng
truyền thông hợp tác. Trong hệ thống này, một nút nguồn truyền thông tin tới một nút đích
thông qua đường truyền trực tiếp hoặc thông qua nút chuyển tiếp được lựa chọn. Giao thức
giải mã và chuyển tiếp (DF: decode and forward) được sử dụng tại nút chuyển tiếp, và kĩ
thuật lựa chọn kết hợp (SC: selection combination) được áp dụng tại nút nguồn để lựa chọn
nút chuyển tiếp tốt nhất. Phẩm chất hệ thống được đánh giá qua xác suất dừng trên kênh có
phân bố Nakagami-m. Phân tích giải tích và các phương trình gần đúng được đề xuất, tính
toán và so sánh với mô phỏng Monte-Carlo. Kết quả mô phỏng tương đồng với kết quả tính
toán, điều này chứng minh tính chính xác của phương pháp tính toán của chúng tôi.
48File đính kèm:
 performance_analysis_of_rf_energy_harvesting_cooperative_com.pdf
performance_analysis_of_rf_energy_harvesting_cooperative_com.pdf

