Nuclear Science and Technology - Volume 9, Number 2, June 2019
Abstract: The paper investigates various computational modelling issues associated with VVER-440
fuel depletion, relevant to burnup credit. The SCALE system and the TRITON sequence are used for the
calculations. The effects of variations in depletion parameters and used calculation methods on the
isotopic vectors are investigated. The burnup behaviour of Gadolinium is quite important in actual core
analysis, but its behaviour is somewhat complicated, requiring special treatment in numerical modelling
and calculations. Therefore, a special part of the paper is devoted to the treatment of Gadolinium-bearing
fuels. Moreover, some discussions on power normalization are also included. To assess the acquired
modelling experience used to predict the VVER-440 spent fuel nuclide composition, the measured
compositions of Novovoronezh NPP irradiated fuel assembly are compared to data calculated by
TRITON sequence. The samples of fuel assembly with 3.6 wt. % U-235 enrichment underwent 4-cycle
campaign of totally 1109 effective full power days in the core and cooling period of 1-13 years.
Calculated concentrations are compared to measured values burdened with their experimental
uncertainties for totally 47 nuclides. The calculated results show overall a good agreement for all
nuclides, differences from measured are pointed out and discussed in the paper.
Keywords: burnup, VVER 440, modelling, SCALE.
I. INTRODUCTION
The prediction accuracy of burnup
calculations is a critical factor in the reactor
analysis sequence. The core properties depend
on the actual composition of the fuel; thus, the
characteristics of the reactor core undergo
changes during burnup. Moreover, the isotopic
composition of the spent fuel discharged from
the core is a key factor in both the operations
and the material control activities of the deep
geological repository. An accurate estimate of
the time-dependent radionuclide inventory in
this material is necessary to evaluate many
spent fuel issues, including neutron and
gamma-ray source terms for shielding analysis,
decay-heat source terms for temperature
distribution and radiological and chemical
toxicity for environmental impact
consideration. Slovakia has four nuclear
reactors generating half of its electricity and
another two under construction. This paper
investigates and summarizes modelling issues
associated with VVER-440 fuel depletion
performed by the SCALE system [1]. The
effects of variations in the depletion parameters
and used calculation methods on the isotopic
vectors are investigated. The burnup behaviour
of Gadolinium, a burnable poison in nuclear
fuel, is quite important in actual core analysis;
therefore, a special part of the paper is devoted
to this issue. Finally, some discussions on
power normalization are also included. Other
publications relevant to burnup modelling
issues for PWR can be found in [2,3]. To
assess the ability of the SCALE system and the
associated nuclear data to predict the VVER-
measured compositions of Novovoronezh NPP
irradiated fuel assembly are compared to data
calculated by the TRITON sequence in the last
part of the paper. The work done follows the
simplified specification of the computational
benchmark based on the #2670 ISTC project
[4] providing VVER-440 data for 8 samples
cut outs of 4 fuel pins of the fuel assembly
(FA) No. D26135 with the average reached
burnup 38.5 MWd/kgU. The #2670 ISTC
project has was carried out between years 2003
and 2005. Measurements of samples cut out of
were performed in RIAR Dimitrovgrad,
Russia, the final project report is publicly
accessible [6]. The FA irradiation was done
during the 15 - 18 core loads in the fourth
power unit of Novovoronezh NPP.

Trang 1
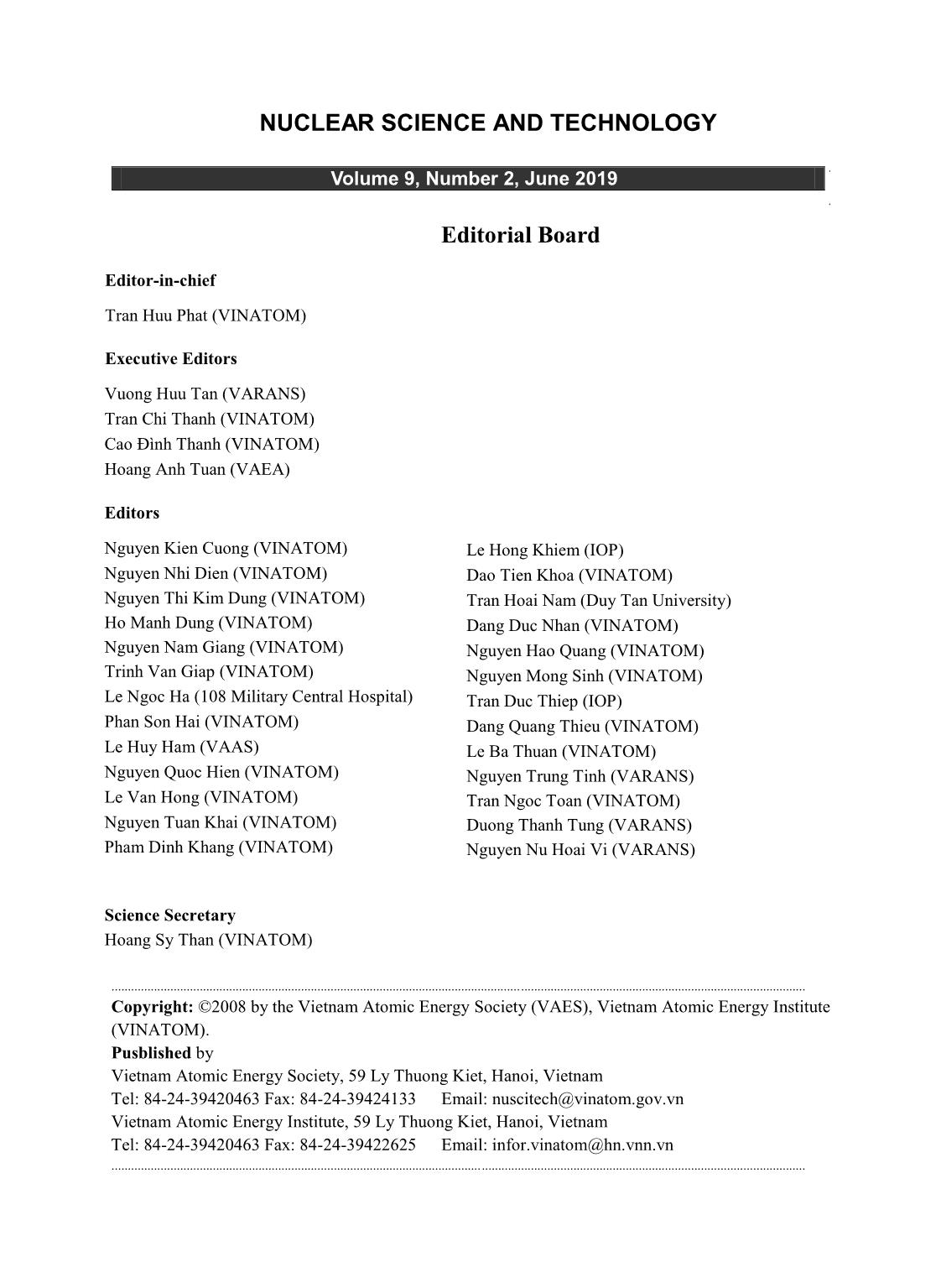
Trang 2

Trang 3

Trang 4

Trang 5

Trang 6

Trang 7
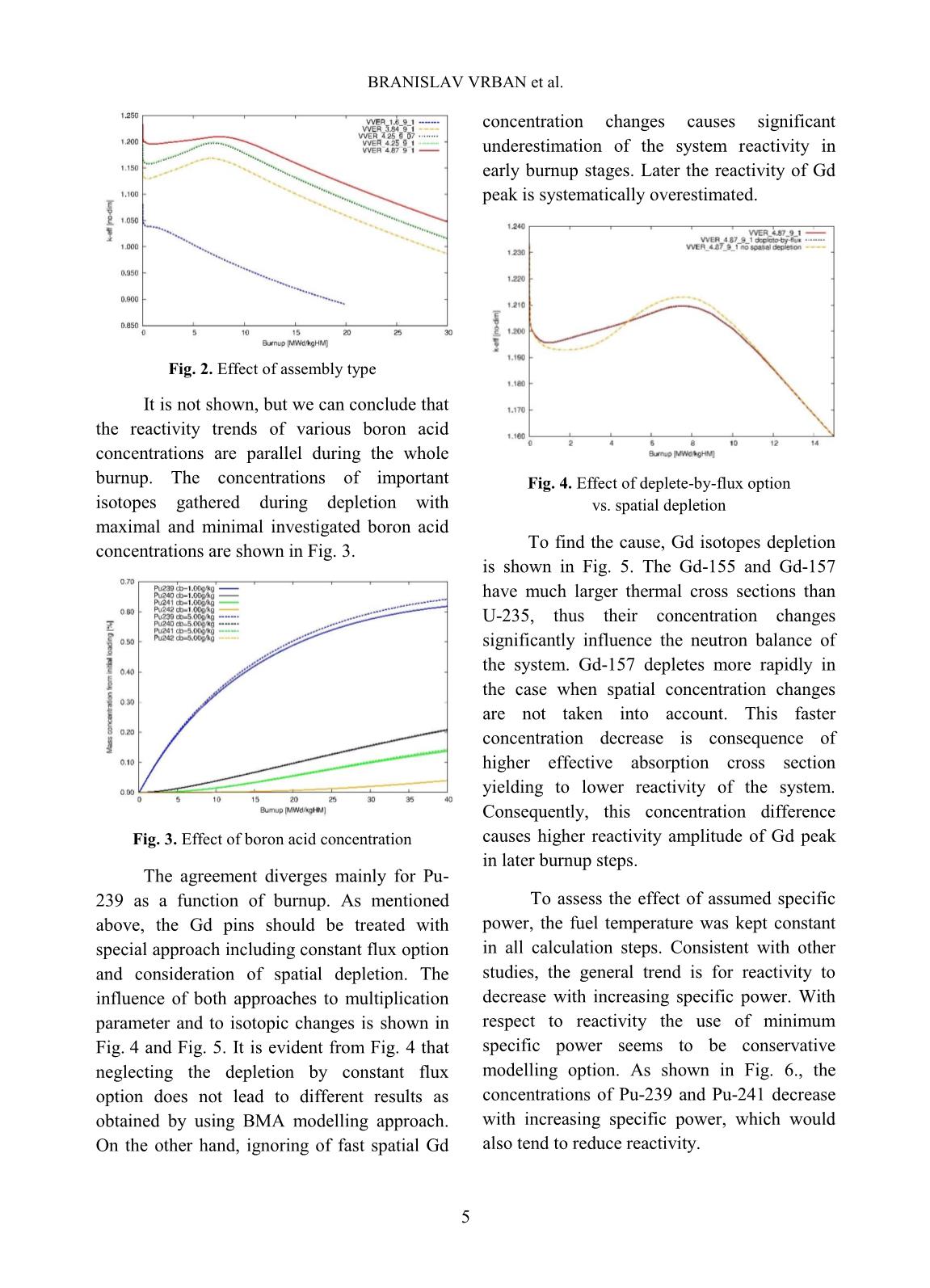
Trang 8
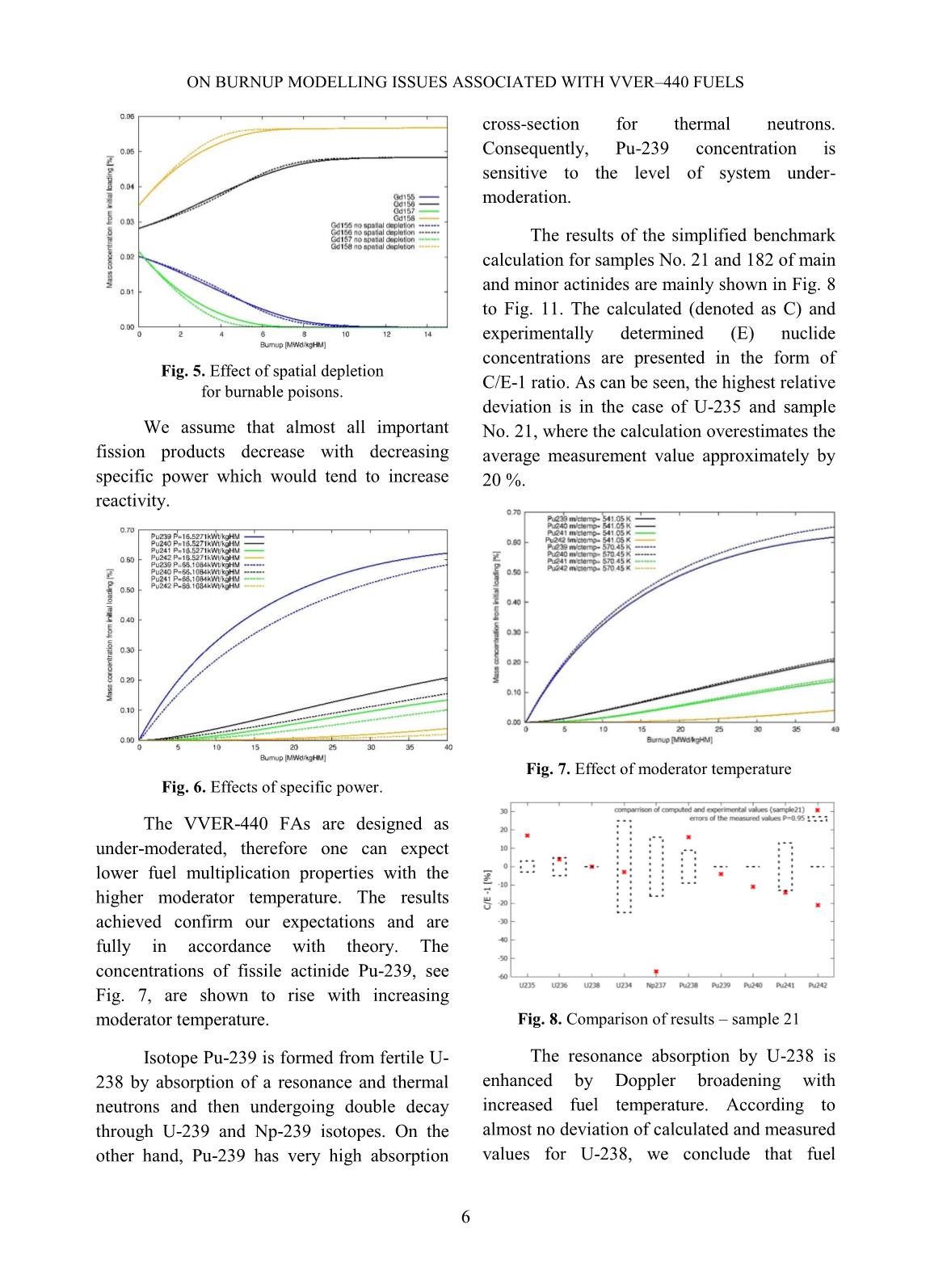
Trang 9

Trang 10
Tải về để xem bản đầy đủ
Tóm tắt nội dung tài liệu: Nuclear Science and Technology - Volume 9, Number 2, June 2019

tem [7]. This paper presents results of applying Simion program to build some design models for the system based on design principles of some built systems in the world and propose a feasible conceptual design of the system that can be used to prepare for the stage of detailed engineering design and construction of the system in the future. II. CONTENTS A. Overview of Simion program Simion is a software package used primarily to model electrostatic and magnetic fields and calculate trajectories of charged particles in these fields when introducing the electrode configuration with voltage and initial conditions of the particles [8]. Simion is intended to provide direct and highly interactive methods for simulating a wide variety of general ion optics problems such as modeling ion source and detector optics, ion traps, quadrupoles, etc. Electrostatic and magnetic fields can be modeled as boundary value problem solutions of a partial differential equation called the Laplace equation (or the Poisson equation). The specific method used within Simion to solve this equation is an over-relaxation technique, which is a finite difference method to obtain a best estimate of potentials for each point within the fields. After the electrostatic and magnetic fields have been obtained, a standard fourth-order Runge-Kutta method is used for numerical integration of the charged particle trajectory in three dimensions based on the particle definition parameters provided by users. In particular, Simion provides functions of extensive support in the definition of geometry, data logging, and visualization. One of the most useful features of Simion is the capability of using user programs inside the program. This feature allows users to directly utilize a variety of programming languages including Lua, C++, C, PRG, etc. to flexibly and efficiently extend simulation capabilities of the program. B. Methods According to several reference system designs, we have successfully built some simulation models that can be possible designs for the system by using Simion. Due to application limitations of the program, the design models are only used to simulate the operating principle of slow positron beam systems rather than specify detailed engineering designs. Three main individual simulation models of the slow positron beam system have been built including: CAO THANH LONG et al. 47 - The straight-shaped model using the ExB filter (figure 1). - The 500 bent-shaped model based on the design of the SPONSOR system [9, 10] (figure 2). - The 900 bent-shaped model based on the design of a slow positron beam system from Martin Luther University Halle- Wittenberg, Germany [11] (figure 3). Fig. 1. The straight-shaped model using ExB filter. Fig. 2. The 50 0 bent-shaped model. Fig. 3. The 90 0 bent-shaped model. Some positron trajectory calculation tests have been performed for each model in order to investigate the influence of design parameters of each model on the quality of positron beam obtained on targets, thereby determining which model was the most reasonable design. The selected model has been proposed as a feasible conceptual design for the future system. Tables of design parameters for the proposed conceptual design include geometry parameters, electric parameters and design parameters of coils creating magnetic fields. CONCEPTUAL DESIGNING OF A SLOW POSITRON BEAM SYSTEM USING 48 C. Results and Discussions Case 1: Simulation of the trajectory of a monoenergetic positron beam Simion has been used to calculate the trajectories for 1000 monoenergetic positrons in a beam emitted isotropically from a circular uniform distribution source with a radius of 2 mm for each model. The initial kinetic energy of the beam was 3 eV. The voltages that were applied to the pre-accelerator and the accelerator of each model were 27 V and 30 kV, respectively. The simulation results are shown below in table I, figure 4 and figure 5. Table I. The simulation results for trajectory of a monoenergetic positron beam Straight-shaped model 50 0 bent-shaped model 90 0 bent-shaped model Total number of positrons from source 1000 1000 1000 Total number of positrons coming to target 789 807 795 The ratio of positron number coming to target to total positron number 78.9% 80.7% 79.5% Beam radius on target 2.75 mm 2.39 mm 2.79 mm Fig. 4. Spatial distribution of positron beam on the target of the straight-shaped model (left), the 50 0 bent- shaped model (middle) and the 90 0 bent-shaped model (right) Fig. 5. Energy distribution of positron beam on the target of the straight-shaped model (left), the 50 0 bent- shaped model (middle) and the 90 0 bent-shaped model (right) (FWHM = 5.50 eV) (FWHM = 1.34 eV) (FWHM = 5.71 eV) CAO THANH LONG et al. 49 From the simulation results, it has been found that the quality of positron beam obtained on the targets is influenced by the model designs. The models with the different designs do not have completely identical uniform magnetic fields along their axes, resulting in different qualities of obtained positron beam on the targets. The results of the beam radius of positron beam on the targets show that obtained positron beam in case of using the 50 0 bent-shaped model has better convergence compared to the others. Furthermore, full width at half maximum (FWHM) of the energy distribution of positron beam on the target for the 50 0 bent-shaped model was smaller than those of the others. It has shown that the obtained positron beam of the 50 0 bent-shaped model was more mono-energetic than those of the others. Case 2: Simulation of the trajectory of a monoenergetic positron beam in case that a solenoid deviated from its original position Another trajectory calculation test has been done for each model with the same monoenergetic positron beam in case that the solenoid surrounding the accelerator of each model deviated from its original position. Distributions of positron beam on the target of each model have been investigated for comparison. We have simulated two situations that the solenoid deviations were 1 cm and 2 cm. The simulation results are shown below in figure 6, figure 7 and figure 8. Fig.6. Spatial distribution of positron beam on the target of the straight-shaped model in case of solenoid deviation of 0 cm (left), 1 cm (middle) and 2 cm (right). Fig.7. Spatial distribution of positron beam on the target of the 50 0 bent-shaped model in case of solenoid deviation of 0 cm (left), 1 cm (middle) and 2 cm (right). CONCEPTUAL DESIGNING OF A SLOW POSITRON BEAM SYSTEM USING 50 Fig. 8. Spatial distribution of positron beam on the target of the 90 0 bent-shaped model in case of solenoid deviation of 0 cm (left), 1 cm (middle) and 2 cm (right). The comparison results have shown that the solenoid deviation influenced the quality of the positron beam that comes to the targets. For the straight-shaped model, there were a few positrons obtained on the target in case of solenoid deviation of 1 cm. In the case of a solenoid deviation of 2 cm, we have even got no positrons coming to the target. For the 90 0 bent-shaped model, the obtained positron beam has deviated much more from the target center compared with that of the 50 0 bent- shaped model. The beam has even distorted its shape when increasing the solenoid deviation to 2 cm. Therefore, we have concluded that the 50 0 bent-shaped model in this simulation case would be the optimal model compared with the others. III. CONCLUSIONS We have come up with the selection of the 50 0 bent-shaped model as a conceptual design for our slow positron beam system based on simulation test results and consideration of the design feasibility of the simulation models. The proposed model can be a good basis for detailed engineering design and construction of the system in the future. The study results have also demonstrated that Simion program is a very suitable tool for modeling and simulation of the trajectory of positron beam flying through electrostatic fields and magnetic fields of a slow positron beam system. Research of searching for other simulation programs to combine with Simion should be done to further optimize the conceptual design. REFERENCES [1]. P. K. Pujari, K. Sudarshan and D. Dutta (Ed.), "11th International Workshop on Positron and Positronium Chemistry (PPC-11)", Journal of Physics: Conference Series, Volume 618, Conference 1, 2015. [2]. P. G. Coleman (Ed.), “Positron Beams and their applications”, World Scientific, Singapore, 2000. [3]. F. A. Selim, A.W. Hunt, J.A. Golovchenko, R. H. Howell, R. Haakenaasen, K.G. Lynn, “Improved source and transport of monoenergetic MeV positrons”, Nuclear Instruments and Methods in Physics Research B 171 (2000), 182-188, 2000. [4]. S. May-Tal Beck, D. Cohen, E. Cohen, A. Kelleher, O. Hen, J. Dumas, E. Piasetzky, N. Pilip, G. Ron, I. Sabo-Napadensky, R Weiss- Babai, “Design of the Slow POsitron faciliTy (SPOT) in Israel”, 13th International Workshop on Slow Positron Beam Techniques and Applications, 2014. CAO THANH LONG et al. 51 [5]. C. K. Cheung, P. S. Naik, C. D. Beling, S. Fung, H. M. Weng, “Performance of a slow positron beam using a hybrid lens design”, Department of Physics, University of Hong Kong, Pokfulam Road, Hong Kong, PR China, 2006. [6]. Xu Hong-Xia, Liu Jian-Dang, Gao Chuan-Bo, Weng Hui-Min, Ye Bang-Jiao, “SIMION simulation of a slow pulsed positron beam”, Department of Modern Physics, University of Science and Technology of China, Hefei 230026, China, 2012. [7]. Cao Thanh Long, Nguyen Trung Hieu, Tran Quoc Dung, Huynh Dong Phuong, “Some initial results of simulating a positron beam system by using SIMION”, Nuclear Science and Technology, Vol.7, No. 3, 17-24, 2017. [8]. David J. Manura, David A. Dahl, “SIMION Version 8.0/8.1 User Manual”, Document Revision 5, Scientific Instrument Services Inc., 2011. [9]. Wolfgang Anwanda, Gerhard Brauer, Maik Butterling, Hans-Rainer Kissener, Andreas Wagner, “Design and Construction of a Slow Positron Beam for Solid and Surface Investigations”, Defect and Diffusion Forum Vol. 331, 2012. [10]. Wolfgang Anwanda, Gerhard Brauer, Hans-Rainer Kissener, “Magnetically guided slow positron beam for defect studies”, Positron Group of TU Dresden at Research Centre Rossendorf, Dresden, Germany, 1994. [11]. R. Krause-Rehberg, “Simple design for a continuous magnetically guided positron beam – and – News from the EPOS project”, Institute of Physics, Martin Luther University Halle-Wittenberg, Germany, 2010. INSTRUCTIONS FOR AUTHORS GENERAL INFORMATION Nuclear Science and Technology (NST), an international journal of the Vietnam Atomic Energy Society (VAES) and Vietnam Atomic Energy Institute (VINATOM), quarterly publishes articles related to theory and application of nuclear science and technology. All papers and technical notes will be refereed. It is understood that the paper has been neither published nor currently submitted for publication elsewhere. The copyright of all published papers and notes will be transferred in VAES. DETAILED FIELDS NST coves all fields of nuclear science and technology for peaceful utilization of nuclear energy and radiation. Authors should choose one of the following fields at the time they submit their manuscript: 1) Nuclear Physics, 2) Nuclear Data, 3) Reactor Physics, 4) Thermal Hydraulics, 5) Nuclear Safety, 6) Nuclear I&C, 7) Nuclear Fuel and Materials, 8) Radioactive Waste Management, 9) Radiation Protection, 10) Radiation Technology, 11) Nuclear Techniques in Food and Agriculture, 12) Nuclear Medicine and Radiotherapy, 13) Nuclear Techniques in Industries, 14) Environment Radioactivity, 15) Isotope Hydrology, 16) Nuclear Analytical Methods, 17) Health Physics, 18) Fusion and Laser Technology. MANUSCRIPT SUBMISSION Manuscript for publication should be submitted to the Editorial Office in triplicate by postal mail. For electronical submission use nuscitech@vinatom.gov.vn. Submission Address Department of Planning, R&D Management Vietnam Atomic Energy Institute, 59 Ly Thuong Kiet Street, Hanoi, Vietnam E-mail: nuscitech@vinatom.gov.vn. MANUSCRIPT PREPARATION Manuscripts must be written in English with adequate margins and indented paragraph. All manuscript must use SI (metric) units in text, figures, and tables. Manuscripts should in general be organized in the following order: title, names of authors and their complete affiliation including zip code, abstract (not exceeding 200 words), keywords (up to 7), introduction, main body of a paper, acknowledgments, references, appendices, table & figure captions, tables and figures. Unnecessary sections may be omitted. Headings: Use I, II, for major headings and A, B, for secondary headings. Mathematical formulas: All mathematical formulas should be clearly written, with special consideration to distinctive legibility of sub-and superscripts. Equation (at least the principal ones) should be numbered consecutively using Arabic numerals in parentheses in the right hand margin. Tables and Figures: Tables should be numbered with Roman numerals. Figures should be numbered consecutively with Arabic numerals in order of their first appearance and have a complete descriptive title. They should be typed on separate sheets. Tables should no repeat data which are available elsewhere in the paper. Figures should be original ink drawing or computer drawn figures in the original and of high quality, ready for direct reproduction. Figures should be referred to in the text as, for example, Fig. 1., or Fig. 2. . Reference: References should be listed at the end of the text and presented as follows: [1] C. Y. Fu et al., Nuclear Data for Science and Technology, S. M. Qaim (Ed.), p. 587 (1991). [2] C. Kalbach, Z. Phys, A283, 401 (1977). [3] S. Shibata, M. Imamura, T. Miyachi and M. Mutou, “Photonuclear spallation reactions in Cu”, Phys. Rev. C 35, 254 (1987). KHOA HỌC VÀ CÔNG NGHỆ HẠT NHÂN Chịu trách nhiệm xuất bản TRẦN HỮU PHÁT Chịu trách nhiệm nội dung TRẦN HỮU PHÁT TRẦN CHÍ THÀNH Trình bày LÊ THÚY MAI In 200 cuốn, khổ 19x26,5cm tại Công ty TNHH Trần Công Địa chỉ: số 12 ngách 155/176 Đường Trường Chinh, Hà Nội Giấy đăng ký kế hoạch xuất bản số: 770/GP-BTTTT cấp ngày 20 tháng 5 năm 2011 In xong và nộp lưu chiểu Quý IV năm 2019 25 000đ
File đính kèm:
 nuclear_science_and_technology_volume_9_number_2_june_2019.pdf
nuclear_science_and_technology_volume_9_number_2_june_2019.pdf

