Burnup calculation of the OECD VVER-1000 LEU benchmark assembly using MCNP6 and SRAC2006

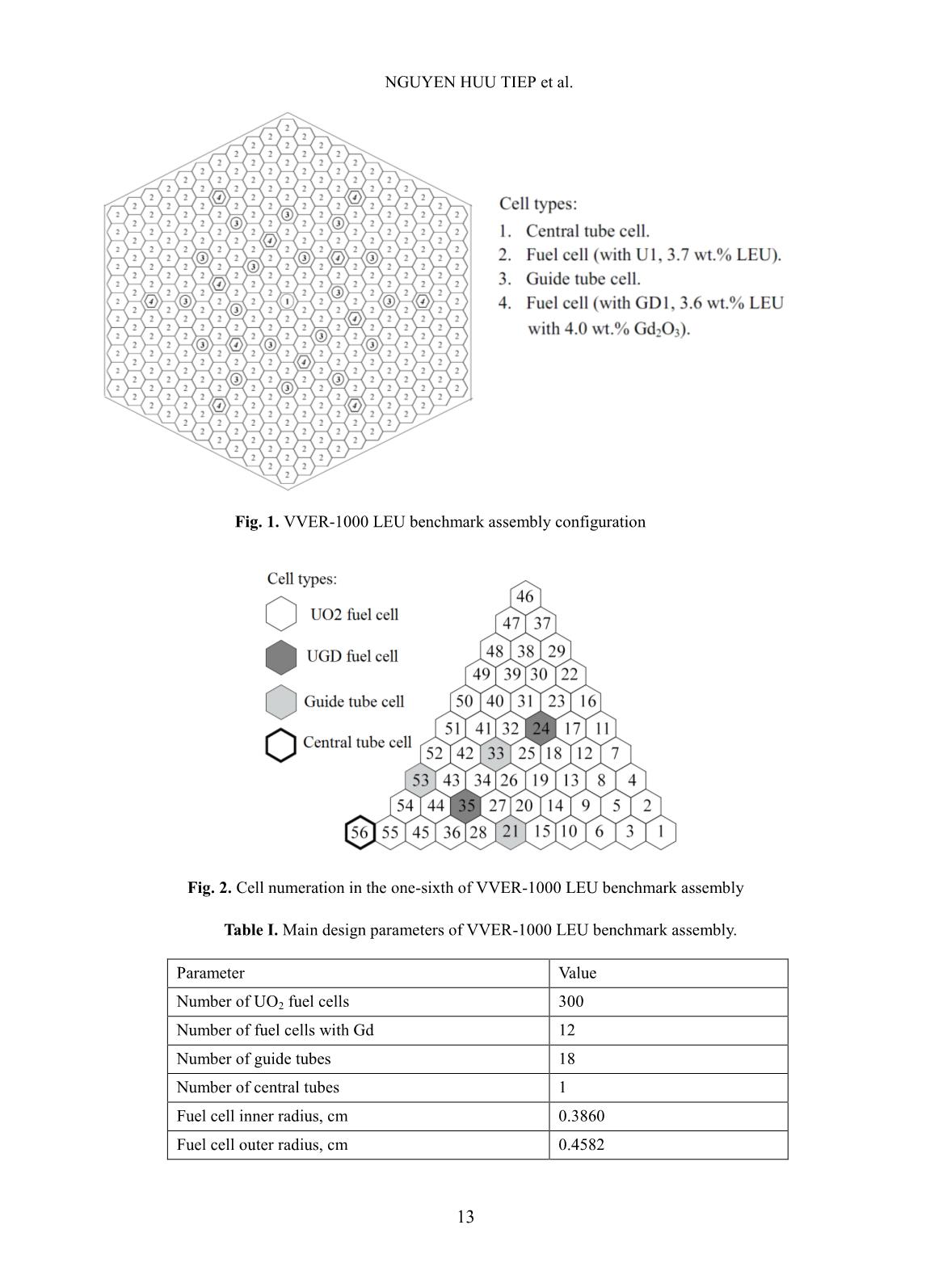
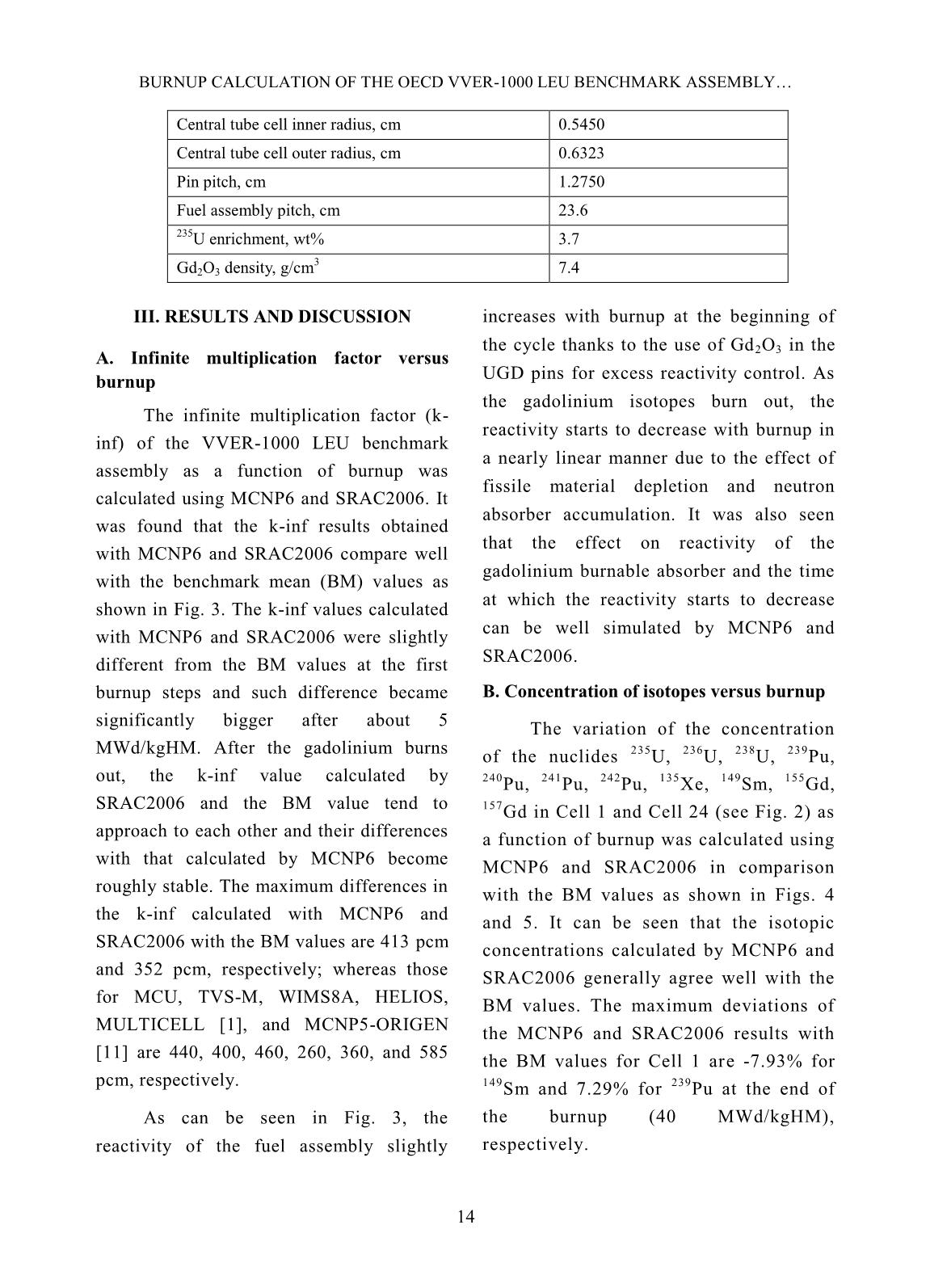
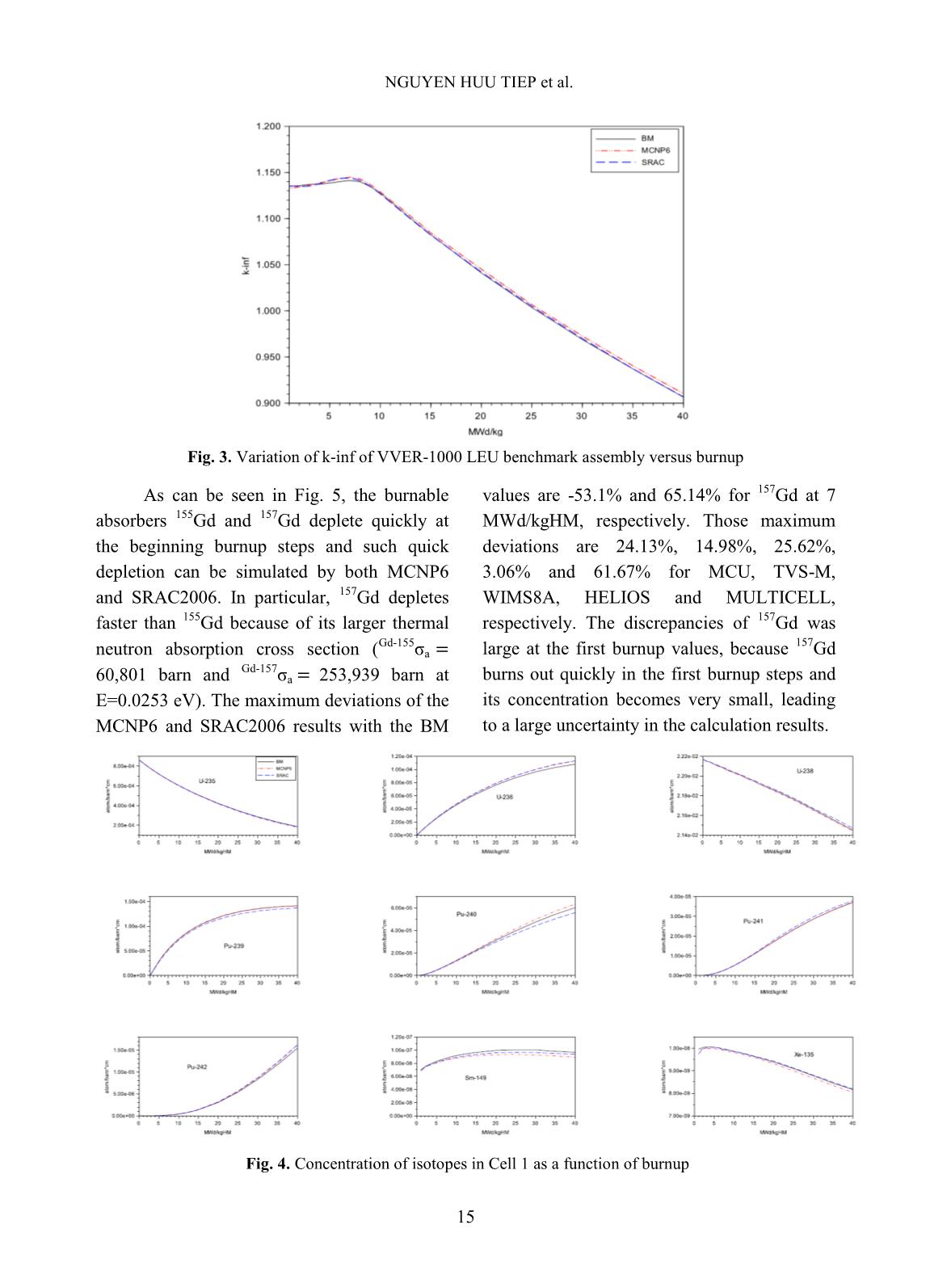
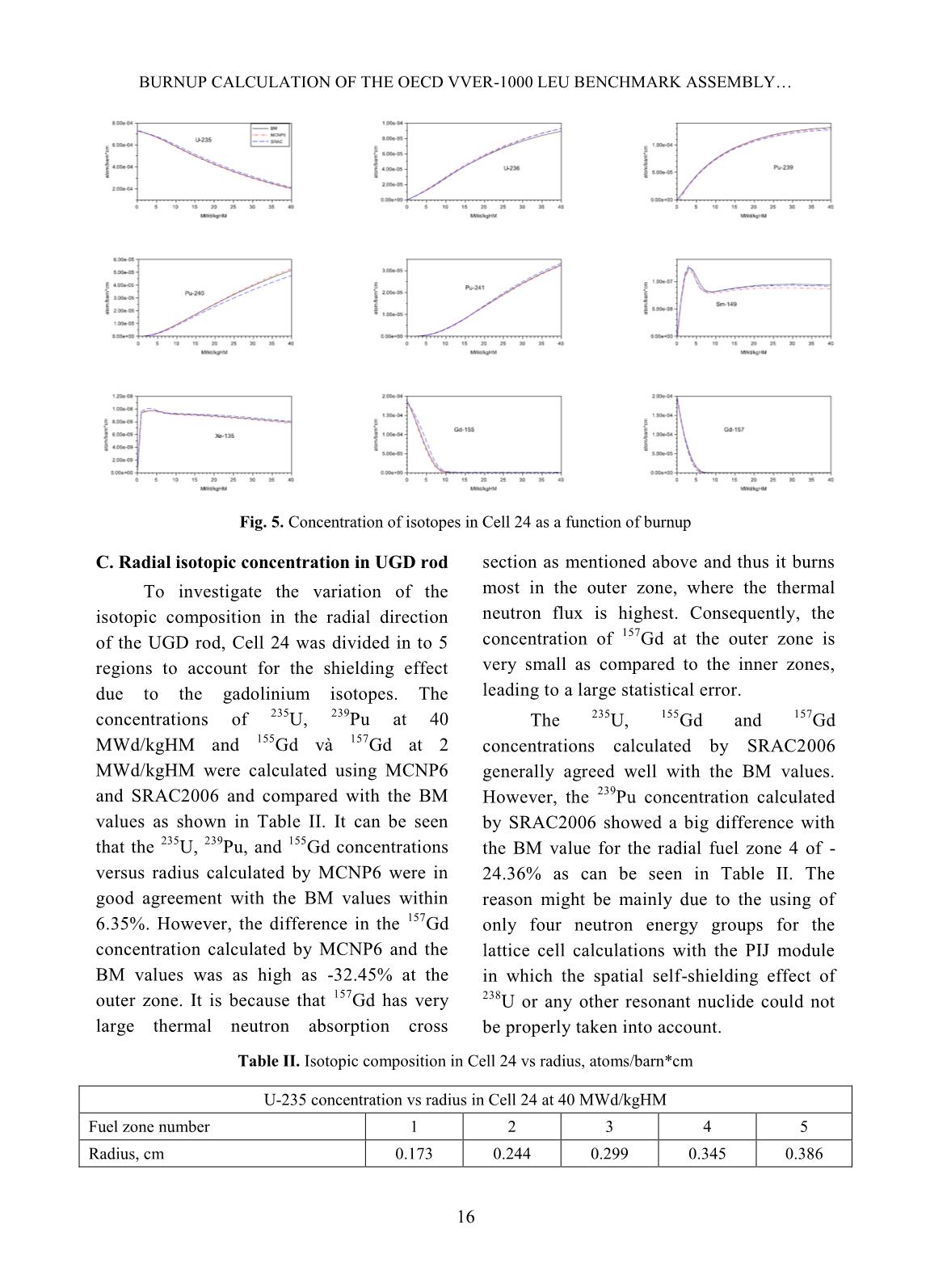
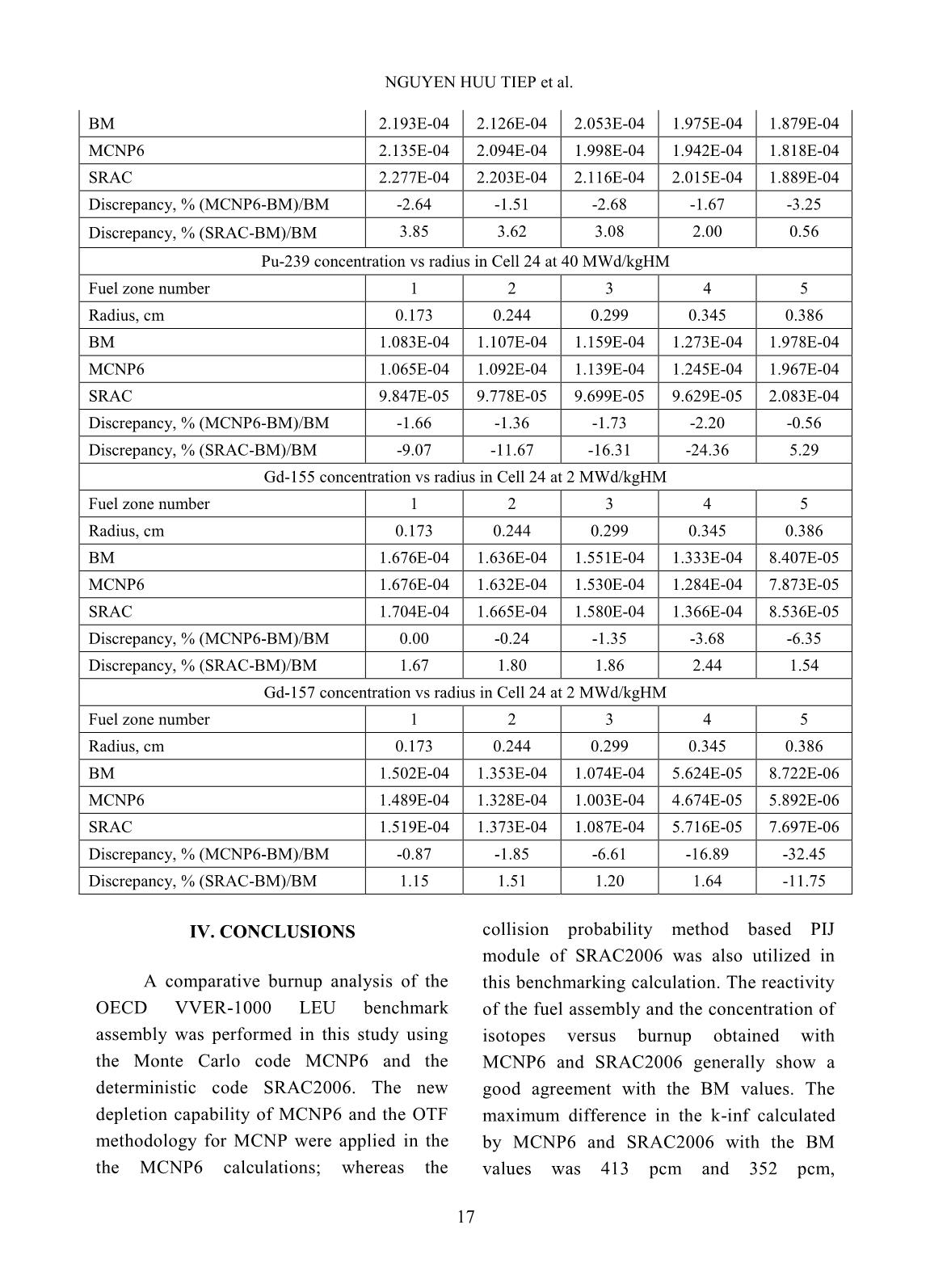
cludes 
the new depletion capability that links steady 
state flux calculations in MCNP6 and nuclide 
depletion calculations in CINDER90. A 
steady state flux calculation is run in MCNP6 
to determine the system eigenvalue, group 
fluxes, energy integrated reaction rates, 
fission multiplicity, and recoverable energy 
per fission. CINDER90 then uses these 
values generated by MCNP6 to perform 
depletion calculation to generate new number 
densities for the next time step. MCNP6 
takes the new number densities generated by 
CINDER90 for the next steady state flux 
calculation. This linked process is repeated 
until the end of the final time step. It is noted 
that CINDER90 contains transmutation data 
information for over 3400 isotopes, 1325 
fission products, yield set for 30 actinides. 
However, the default nuclear data 
in MCNP6 are given at certain 
temperatures for heavy isotopes (293.6, 
600, 900, 1200 and 2500K); while the 
fuel temperature of 1027K is needed in 
this calculation. There are various 
methods to cope with such kind of 
temperature dependence [14]. One of the 
suitable methods for MCNP6 is the OTF 
methodology for fitting of Doppler 
broadened cross sections and this method 
was applied in this study. The OTF data 
for heavy isotopes in the VVER-1000 
LEU benchmark assembly that 
correspond to the temperature range of 
293.6 to 1200 K were created from the 
ENDF/B-VII.0 library at the temperature 
of 293.6 K. 
The PIJ module with its cell burnup 
routine of the SRAC2006 code system was 
also utilized for the burnup calculation of 
the VVER-1000 LEU benchmark assembly. 
The PIJ module that is based on the 
collision probability method was used for 
lattice cell calculations. The cell burnup 
routine used one-group collapsed flux 
distribution and the collapsed microscopic 
cross sections to solve the depletion 
equation using the Bateman’s method. The 
burnup calculation using the cell burnup 
routine of the PIJ module was performed 
with 40 steps of 0.25 MWd/kgHM followed 
by 5 steps of 1.0 MWd/kgHM and 10 steps 
of 2.5 MWd/kgHM. The 107 neutron energy 
groups based on the ENDF/B-VII.0 library 
were collapsed to four groups for use in the 
SRAC2006 calculations. 
 NGUYEN HUU TIEP et al. 
13 
Fig. 1. VVER-1000 LEU benchmark assembly configuration 
Fig. 2. Cell numeration in the one-sixth of VVER-1000 LEU benchmark assembly 
Table I. Main design parameters of VVER-1000 LEU benchmark assembly. 
Parameter Value 
Number of UO2 fuel cells 300 
Number of fuel cells with Gd 12 
Number of guide tubes 18 
Number of central tubes 1 
Fuel cell inner radius, cm 0.3860 
Fuel cell outer radius, cm 0.4582 
BURNUP CALCULATION OF THE OECD VVER-1000 LEU BENCHMARK ASSEMBLY 
14 
Central tube cell inner radius, cm 0.5450 
Central tube cell outer radius, cm 0.6323 
Pin pitch, cm 1.2750 
Fuel assembly pitch, cm 23.6 
235U enrichment, wt% 3.7 
Gd2O3 density, g/cm
3
 7.4 
III. RESULTS AND DISCUSSION 
A. Infinite multiplication factor versus 
burnup 
The infinite multiplication factor (k-
inf) of the VVER-1000 LEU benchmark 
assembly as a function of burnup was 
calculated using MCNP6 and SRAC2006. It 
was found that the k-inf results obtained 
with MCNP6 and SRAC2006 compare well 
with the benchmark mean (BM) values as 
shown in Fig. 3. The k-inf values calculated 
with MCNP6 and SRAC2006 were slightly 
different from the BM values at the first 
burnup steps and such difference became 
significantly bigger after about 5 
MWd/kgHM. After the gadolinium burns 
out, the k-inf value calculated by 
SRAC2006 and the BM value tend to 
approach to each other and their differences 
with that calculated by MCNP6 become 
roughly stable. The maximum differences in 
the k-inf calculated with MCNP6 and 
SRAC2006 with the BM values are 413 pcm 
and 352 pcm, respectively; whereas those 
for MCU, TVS-M, WIMS8A, HELIOS, 
MULTICELL [1], and MCNP5-ORIGEN 
[11] are 440, 400, 460, 260, 360, and 585 
pcm, respectively. 
As can be seen in Fig. 3, the 
reactivity of the fuel assembly slightly 
increases with burnup at the beginning of 
the cycle thanks to the use of Gd2O3 in the 
UGD pins for excess reactivity control. As 
the gadolinium isotopes burn out, the 
reactivity starts to decrease with burnup in 
a nearly linear manner due to the effect of 
fissile material depletion and neutron 
absorber accumulation. It was also seen 
that the effect on reactivity of the 
gadolinium burnable absorber and the time 
at which the reactivity starts to decrease 
can be well simulated by MCNP6 and 
SRAC2006. 
B. Concentration of isotopes versus burnup 
The variation of the concentration 
of the nuclides 
235
U, 
236
U, 
238
U, 
239
Pu, 
240
Pu, 
241
Pu, 
242
Pu, 
135
Xe, 
149
Sm, 
155
Gd, 
157
Gd in Cell 1 and Cell 24 (see Fig. 2) as 
a function of burnup was calculated using 
MCNP6 and SRAC2006 in comparison 
with the BM values as shown in Figs. 4 
and 5. It can be seen that the isotopic 
concentrations calculated by MCNP6 and 
SRAC2006 generally agree well with the 
BM values. The maximum deviations of 
the MCNP6 and SRAC2006 results with 
the BM values for Cell 1 are -7.93% for 
149
Sm and 7.29% for 
239
Pu at the end of 
the burnup (40 MWd/kgHM), 
respectively. 
 NGUYEN HUU TIEP et al. 
15 
Fig. 3. Variation of k-inf of VVER-1000 LEU benchmark assembly versus burnup 
As can be seen in Fig. 5, the burnable 
absorbers 
155
Gd and 
157
Gd deplete quickly at 
the beginning burnup steps and such quick 
depletion can be simulated by both MCNP6 
and SRAC2006. In particular, 
157
Gd depletes 
faster than 
155
Gd because of its larger thermal 
neutron absorption cross section (
Gd-155σa 
60,801 barn and 
Gd-157σa 253,939 barn at 
E=0.0253 eV). The maximum deviations of the 
MCNP6 and SRAC2006 results with the BM 
values are -53.1% and 65.14% for 
157
Gd at 7 
MWd/kgHM, respectively. Those maximum 
deviations are 24.13%, 14.98%, 25.62%, 
3.06% and 61.67% for MCU, TVS-M, 
WIMS8A, HELIOS and MULTICELL, 
respectively. The discrepancies of 
157
Gd was 
large at the first burnup values, because 
157
Gd 
burns out quickly in the first burnup steps and 
its concentration becomes very small, leading 
to a large uncertainty in the calculation results. 
 Fig. 4. Concentration of isotopes in Cell 1 as a function of burnup 
BURNUP CALCULATION OF THE OECD VVER-1000 LEU BENCHMARK ASSEMBLY 
16 
Fig. 5. Concentration of isotopes in Cell 24 as a function of burnup 
C. Radial isotopic concentration in UGD rod 
To investigate the variation of the 
isotopic composition in the radial direction 
of the UGD rod, Cell 24 was divided in to 5 
regions to account for the shielding effect 
due to the gadolinium isotopes. The 
concentrations of 
235
U, 
239
Pu at 40 
MWd/kgHM and 
155
Gd và 
157
Gd at 2 
MWd/kgHM were calculated using MCNP6 
and SRAC2006 and compared with the BM 
values as shown in Table II. It can be seen 
that the 
235
U, 
239
Pu, and 
155
Gd concentrations 
versus radius calculated by MCNP6 were in 
good agreement with the BM values within 
6.35%. However, the difference in the 
157
Gd 
concentration calculated by MCNP6 and the 
BM values was as high as -32.45% at the 
outer zone. It is because that 
157
Gd has very 
large thermal neutron absorption cross 
section as mentioned above and thus it burns 
most in the outer zone, where the thermal 
neutron flux is highest. Consequently, the 
concentration of 
157
Gd at the outer zone is 
very small as compared to the inner zones, 
leading to a large statistical error. 
The 
235
U, 
155
Gd and 
157
Gd 
concentrations calculated by SRAC2006 
generally agreed well with the BM values. 
However, the 
239
Pu concentration calculated 
by SRAC2006 showed a big difference with 
the BM value for the radial fuel zone 4 of -
24.36% as can be seen in Table II. The 
reason might be mainly due to the using of 
only four neutron energy groups for the 
lattice cell calculations with the PIJ module 
in which the spatial self-shielding effect of 
238
U or any other resonant nuclide could not 
be properly taken into account. 
Table II. Isotopic composition in Cell 24 vs radius, atoms/barn*cm 
U-235 concentration vs radius in Cell 24 at 40 MWd/kgHM 
Fuel zone number 1 2 3 4 5 
Radius, cm 0.173 0.244 0.299 0.345 0.386 
 NGUYEN HUU TIEP et al. 
17 
BM 2.193E-04 2.126E-04 2.053E-04 1.975E-04 1.879E-04 
MCNP6 2.135E-04 2.094E-04 1.998E-04 1.942E-04 1.818E-04 
SRAC 2.277E-04 2.203E-04 2.116E-04 2.015E-04 1.889E-04 
Discrepancy, % (MCNP6-BM)/BM -2.64 -1.51 -2.68 -1.67 -3.25 
Discrepancy, % (SRAC-BM)/BM 3.85 3.62 3.08 2.00 0.56 
Pu-239 concentration vs radius in Cell 24 at 40 MWd/kgHM 
Fuel zone number 1 2 3 4 5 
Radius, cm 0.173 0.244 0.299 0.345 0.386 
BM 1.083E-04 1.107E-04 1.159E-04 1.273E-04 1.978E-04 
MCNP6 1.065E-04 1.092E-04 1.139E-04 1.245E-04 1.967E-04 
SRAC 9.847E-05 9.778E-05 9.699E-05 9.629E-05 2.083E-04 
Discrepancy, % (MCNP6-BM)/BM -1.66 -1.36 -1.73 -2.20 -0.56 
Discrepancy, % (SRAC-BM)/BM -9.07 -11.67 -16.31 -24.36 5.29 
Gd-155 concentration vs radius in Cell 24 at 2 MWd/kgHM 
Fuel zone number 1 2 3 4 5 
Radius, cm 0.173 0.244 0.299 0.345 0.386 
BM 1.676E-04 1.636E-04 1.551E-04 1.333E-04 8.407E-05 
MCNP6 1.676E-04 1.632E-04 1.530E-04 1.284E-04 7.873E-05 
SRAC 1.704E-04 1.665E-04 1.580E-04 1.366E-04 8.536E-05 
Discrepancy, % (MCNP6-BM)/BM 0.00 -0.24 -1.35 -3.68 -6.35 
Discrepancy, % (SRAC-BM)/BM 1.67 1.80 1.86 2.44 1.54 
Gd-157 concentration vs radius in Cell 24 at 2 MWd/kgHM 
Fuel zone number 1 2 3 4 5 
Radius, cm 0.173 0.244 0.299 0.345 0.386 
BM 1.502E-04 1.353E-04 1.074E-04 5.624E-05 8.722E-06 
MCNP6 1.489E-04 1.328E-04 1.003E-04 4.674E-05 5.892E-06 
SRAC 1.519E-04 1.373E-04 1.087E-04 5.716E-05 7.697E-06 
Discrepancy, % (MCNP6-BM)/BM -0.87 -1.85 -6.61 -16.89 -32.45 
Discrepancy, % (SRAC-BM)/BM 1.15 1.51 1.20 1.64 -11.75 
IV. CONCLUSIONS 
A comparative burnup analysis of the 
OECD VVER-1000 LEU benchmark 
assembly was performed in this study using 
the Monte Carlo code MCNP6 and the 
deterministic code SRAC2006. The new 
depletion capability of MCNP6 and the OTF 
methodology for MCNP were applied in the 
the MCNP6 calculations; whereas the 
collision probability method based PIJ 
module of SRAC2006 was also utilized in 
this benchmarking calculation. The reactivity 
of the fuel assembly and the concentration of 
isotopes versus burnup obtained with 
MCNP6 and SRAC2006 generally show a 
good agreement with the BM values. The 
maximum difference in the k-inf calculated 
by MCNP6 and SRAC2006 with the BM 
values was 413 pcm and 352 pcm, 
BURNUP CALCULATION OF THE OECD VVER-1000 LEU BENCHMARK ASSEMBLY 
18 
respectively; while at the end of burnup 
(40 MWd/kgHM) the deviations in the 
nuclide concentrations calculated by 
MCNP6 and SRAC2006 with the BM 
values were generally within -7.93%. It 
was also found that the effect on the 
reactivity of the gadolinium burnable 
absorber and the depletion of the 
155
Gd 
and 
157
Gd isotopes at the beginning of the 
fuel cycle can be well simulated using 
MCNP6 and SRAC2006. 
The isotopic composition variation of 
nuclides 
235
U, 
239
Pu, 
155
Gd and 
157
Gd as a 
function of radii of annular regions of the UGD 
rod was also calculated with MCNP6 and 
SRAC2006 and compared with the BM results. 
They were found to be generally in good 
agreement; however, the SRAC2006 results 
showed a large discrepancy with the BM 
values for the 
239
Pu concentration that might be 
mainly due to the using of only four neutron 
energy groups in the SRAC2006 calculations. 
Consequently, it is highly recommended that 
MCNP6 and SRAC2006 can be used for 
burnup calculation of VVER-1000 fuel 
assemblies. Further investigation of the burnup 
calculation using MCNP6 and SRAC2006 at 
the full core level and MOX core of the 
VVER-1000 reactor is being planned. 
REFERENCE 
[1]. M. Kalugin, et al., A VVER-1000 LEU and 
MOX Assembly Computational Benchmark, 
Nuclear Energy Agency, NEA/NSC/DOC 10, 
2002. 
[2]. L. Thilagam, C. S. Sunny, V. Jagannathan, K. 
V. Subbaiah, “A VVER-1000 LEU and MOX 
assembly computational benchmark analysis 
using the lattice burnup code EXCEL”, Annals 
of Nuclear Energy 36 (2009) 505-519. 
[3]. N. Petrov, G. Todorova, N. P. Kolev, “APOLLO2 
and TRIPOLI4 solutions of the OECD VVER-
1000 LEU and MOX assembly benchmark”, 
Annals of Nuclear Energy 55 (2013) 93–107. 
[4]. M. Zheng, W. Tian, H. Wei, D. Zhang, Y. Wu, 
S. Qiu, G. Su, “Development of a MCNP–
ORIGEN burn-up calculation code system and 
its accuracy assessment”, Annals of Nuclear 
Energy 63 (2014) 491–498. 
[5]. L. Mercatali, A. Venturini, M. Daeubler, V. H. 
Sanchez, “SCALE and SERPENT solutions of 
the OECD VVER-1000 LEU and MOX 
burnup computational benchmark”, Annals of 
Nuclear Energy, 83 (2015) 328-341. 
[6]. H. K. Louis, E. Amin, “The Effect of burnup 
on reactivity for VVER-1000 with MOXGD 
and UGD fuel assemblies using MCNPX 
code”, Journal of Nuclear and Particle Physics 
2016, 6 (3) 61-71. 
[7]. S. A. Khan, V. Jagannathan, U. Kannan, A. 
Mathur, “Study of VVER-1000 OECD LEU 
and MOX computational benchmark with 
VISWAM code system”, Nuclear Energy and 
Technology 2 (2016) 312-334. 
[8]. L. B. Vien, L. V. Vinh, H. T. Nghiem, N. K. 
Cuong, “Design analyses for full core 
conversion of the Dalat nuclear research 
reactor”, Nuclear Science and Technology, Vol. 
4, No. 1, p.10-25, March 2014. 
[9]. N. N. Dien, L. B. Vien, L. V. Vinh, H. T. 
Nghiem, N. K. Cuong, “Full core conversion 
and operational experience with LEU fuel of 
the Dalat nuclear research reactor”, RERTR 
2014 - 35
th
 International Meeting on Reduced 
Enrichment for Research and Test Reactors, 
IAEA Vienna Interational Center, Vienna, 
Austria, October 2014. 
[10]. N. K. Cuong, H. T. Nghiem, V. H. Tan, “The 
Development of depletion program coupled 
with Monte Carlo computer code”, The 11th 
 NGUYEN HUU TIEP et al. 
19 
National Conference on Nuclear Science and 
Technology, Da Nang,Vietnam, August 2015. 
[11]. N. H. Hiep, N. H. Tiep, T. V. Phu, N. T. Khai, 
“Development of an MCNP5-ORIGEN2 
coupling scheme for burnup calculation of 
VVER-1000 fuel assemblies”, Nuclear Science 
and Technology, Vol. 6, No. 3 (2016), pp. 16-30. 
[12]. D. B. Pelowitz et al., MCNP6TM User’s Manual, 
Version 1.0, LA-CP-13-00634, Rev. 0, 2013. 
[13]. K. Okumura, T. Kugo, K. Kaneko, and K. 
Tsuchihashi, SRAC2006: A Comprehensive 
Neutronics Calculation Code System, JAEA-
Data/Code 2007-004, 2007. 
[14]. F. Brown, W. Martin, G. Yesilyurt, S. 
Wilderman, On-The-Fly Neutron Doppler 
Broadening for MCNP, Los Alamos 
National Laboratory, LA-UR-12-00700, 
2012.