Dyson equations for green functions of electrons in open single - level quantum dot

ontains the Green functions Hcc¯σσ′(t− t′), Gac¯σσ′(k; t− t′) and Gbc¯σσ′(k; t− t′). These
new functions must satisfy following differential equations which can be also derived by
using the equations of motion (11)–(14)[
i
d
dt
− (E + U)
]
Hcc¯σσ′(t − t′)
=nδC(t− t′) +
∑
k
[V ∗a (k)H
ac¯
σσ′(k; t− t′) + V ∗b (k)Hbc¯σσ′(k; t− t′)]
+
∑
k
[
V ∗a (k)G
acc¯c¯
σσ′ (k; t− t′) + V ∗b (k)Gbcc¯c¯σσ′ (k; t− t′)
− Va(k)Gcca¯c¯σσ′ (k; t− t′)− Vb(k)Gccb¯c¯σσ′ (k; t− t′)
]
,
(16)
where
n =
〈
c+↑ c↑
〉
=
〈
c+↓ c↓
〉
, (17)[
i
d
dt
− Ea(k)
]
Gac¯σσ′(k; t− t′) = Va(k)Gcc¯σσ′(t− t′), (18)
and similarly for Gbc¯σσ′(k; t− t′).
Introduce the complex-time Green function SE(t − t′) of the free electron with a
given energy E. It is the solution of the differential equation[
i
d
dt
− E
]
SE(t− t′) = δC(t − t′). (19)
Then we can write the solution of the differential equation (18) in the integral form
Gac¯σσ′(k; t− t′) = Va(k)
∫
C
dt′′SE(t− t′′)Gcc¯σσ′(t− t′ ′), (20)
and similarly for Gbc¯σσ′(k; t−t′). Substituting the expression of the form (20) for Gac¯σσ′(k; t−
t′) and Gbc¯σσ′(k; t − t′) into the r.h.s. of the differential equation (15) for Gcc¯σσ′(t − t′) we
rewrite this equation in the new form[
i
d
dt
− E
]
Gcc¯σσ′(t−t′) = δσσ′δC(t−t′)+UHcc¯σσ′(t−t′)+
∫
C
dt′′Σ(1)(t− t′′)Gcc¯σσ′(t′′ − t), (21)
Dyson Equations for Green Functions of Electrons in Open Single-level Quantum Dot 5
where Σ(1)(t − t′′) is the following self-energy part
Σ(1)(t− t′) =
∑
k
{
|Va(k)|2 SEa(k)(t − t′) + |Vb(k)|2 SEb(k)(t− t′)
}
. (22)
The differential equation for the Green function Hcc¯σσ′(t − t′) contains the Green
function Hac¯σσ′(k; t− t′), Hbc¯σσ′(k; t− t′), Gacc¯c¯σσ′ (k; t− t′), Gbcc¯c¯σσ′ (k; t− t′), Gcca¯c¯σσ′ (k; t− t′) and
Gccb¯c¯σσ′ (k; t− t′) which must satisfy following differential equations
[
i
d
dt
−Ea(k)
]
Hac¯σσ′(k; t− t′) = Va(k)Hcc¯σσ′(t− t′), (23)
and similarly for Hbc¯σσ′(k; t− t′),
[
i
d
dt
− Ea(k)
]
Gacc¯c¯σσ′ (k; t− t′)
=
〈{
a−σ(k)cσc+−σ, c
+
σ′
}〉
δC(t− t′) + Va(k)[Hcc¯σσ′(t − t′)−Gcc¯σσ′(t− t′)]
+
∑
l
[V ∗a (l)G
aac¯c¯
σσ′ (k,l; t− t′) + V ∗b (l)Gabc¯c¯σσ′ (k,l; t− t′)]
−
∑
l
[Va(l)Gaca¯c¯σσ′ (k,l; t− t′) + Vb(l)Gacb¯c¯σσ′ (k,l; t− t′)],
(24)
and similarly for Gbcc¯c¯σσ′ (k; t− t′),
{
i
d
dt
− [2E −Ea(k) + U ]
}
Gcca¯c¯σσ′ (k; t− t′)
=
〈{c−σcσa+−σ(k), c+σ′}〉 δC(t− t′)− V ∗a (k)[Hcc¯σσ′(t− t′)−Gcc¯σσ′(t− t′)]
+
∑
l
{
V ∗a (l)[G
aca¯c¯
σσ′ (l,k; t− t′) +Gcaa¯c¯σσ′ (l,k; t− t′)]
+V ∗b (l)[G
bca¯c¯
σσ′ (l,k; t− t′) +Gcba¯c¯σσ′ (l,k; t− t′)]
}
,
(25)
and similarly for Gccb¯c¯σσ′ (k; t− t′). The solutions of the differential equations (23), (24) and
(25) can be written in the integral form
Hac¯σσ′(k; t− t′) = Va(k)
∫
C
dt′′SEa(k)(t− t′ ′)Hcc¯σσ′(t′′ − t′), (26)
6 Nguyen Van Hieu, Nguyen Bich Ha, and Nguyen Van Hop
and similarly for Hbc¯σσ′(k; t− t′),
Gac c¯c¯σσ′ (k; t− t′)
=
〈{a−σ(k)cσc+−σ, c+σ′}〉SEa(k)(t− t′)
+ Va(k)
∫
C
dt”SEa(k)(t − t′′)[Hcc¯σσ′(t− t′)− Gcc¯σσ′(t− t′)]
+
∫
C
dt′′SEa(k)(t− t′′)
∑
l
[V ∗a (l)G
aac¯c¯
σσ′ (k,l; t
′′ − t′) + V ∗b (l)Gabc¯c¯σσ′ (k,l; t′′ − t′)]
−
∫
C
dt′′SEa(k)(t− t′′)
∑
l
[Va(l)Gaca¯c¯σσ′ (k,l; t
′′ − t′) + Vb(l)Gacb¯c¯σσ′ (k,l; t′′ − t′)],
(27)
and similarly for Gbcc¯c¯σσ′ (k; t− t′),
Gcca¯c¯σσ′ (k; t− t′)
=
〈{c−σcσa+−σ (k), c+σ′}〉S2E+U−Ea(k)(t− t′)
− V ∗a (k)
∫
C
dt′′S2E+U−Ea(k)(t− t′′)[Hcc¯σσ′(t′′ − t)−Gcc¯σσ′(t′′ − t)]
+
∫
C
dt′′S2E+U−Ea(k)(t− t′′)
∑
l
{
V ∗a (l)[G
aca¯c¯
σσ′ (l,k; t
′′ − t′) +Gcaa¯c¯σσ′ (l,k; t′′ − t′)]
+V ∗b (l)[G
bca¯c¯
σσ′ (l,k; t
′′ − t′) +Gcba¯c¯σσ′ (l,k; t′′− t′]
}
,
(28)
and similarly for Gccb¯c¯σσ′ (k; t − t′). Substituting these solutions into the r.h.s. of the differ-
ential equation for Hcc¯σσ′(t− t′) we rewrite this equation in the new form
[
i
d
dt
− (E + U)
]
Hcc¯σσ′(t− t′)
=nδσσ′ δC(t− t′) +
∫
C
dt′′Σ(1)(t− t′′)Hcc¯σσ′(t′′ − t)
+
∑
k
{
V ∗a (k)
〈{a−σ(k)cσc+−σ , c+σ′}〉SEa(k)(t − t′)
+ |Va(k)|2
∫
C
dt′′SEa(k)(t− t′′)[Hcc¯σσ′(t− t′)−Gcc¯σσ′(t− t′)]
}
Dyson Equations for Green Functions of Electrons in Open Single-level Quantum Dot 7
+
∫
C
dt′′
∑
k
V ∗a (k)S
Ea(k)(t− t′′)
∑
l
[V ∗a (l)G
aac¯c¯
σσ′ (k,l; t
′′ − t′) + V ∗b (l)Gabc¯c¯σσ′ (k,l; t′′ − t′)]
−
∫
C
dt”
∑
k
V ∗a (k)S
Ea(k)(t − t′′)
∑
l
[Va(l)Gaca¯c¯σσ′ (k,l; t
′′ − t′) + Vb(l)Gacb¯c¯σσ′ (k,l; t′′ − t′)]
−
∑
k
{
Va(k)
〈{c−σcσa+−σ(k), c+σ′}〉S2E+U−Ea(k)(t− t′)
+ |Va(k)|2
∫
C
dt′′S2E+U−Ea(k)(t− t′′)[Hcc¯σσ′(t′′ − t)− Gcc¯σσ′(t′′ − t)]
}
−
∫
C
dt′′
∑
k
Va(k)S2E+U−Ea(k)(t− t′′)
∑
l
V ∗a (l)[G
aca¯c¯
σσ′ (l,k; t
′′ − t′) +Gcaa¯c¯σσ′ (l,k; t′′ − t′)]
−
∫
C
dt′′
∑
k
Va(k)S2E+U−Ea(k)(t− t′′)V ∗b (l)[Gbca¯c¯σσ′ (l,k; t′′ − t′) + Gcba¯c¯σσ′ (l,k; t′′ − t′)]
+ similar terms with the interchange(a↔ b).
(29)
4. MEAN- FIELD APPROXIMATION
The r.h.s. of the equation (29) for the Green function Hcc¯σσ′(t − t′) contains the
multi-electron Green functions
Gaac¯c¯σσ′ (k, l; t− t′), Gabc¯c¯σσ′ (k, l; t− t′), Gaca¯c¯σσ′ (k, l; t− t′), Gacb¯c¯σσ′ (k; t− t′),
Gcaa¯c¯σσ′ (k, l; t− t′), Gbca¯c¯σσ′ (k, l; t− t′), Gbca¯c¯σσ′ (k, l; t− t′)
and the similarly ones with the interchange (a↔ b). In order to decouple this equation
with those for the last multi-electron Green functions we apply the mean-field approx-
imation to the products of four operators in the expressions of above-mentioned Green
functions. Consider first the Green function Gaca¯c¯σσ′ (k, l; t − t′) expressing in terms of the
mean value of the product a¯−σ(k, t)a−σ(l, t)cσ(t)c¯σ′(t′). This product is that of two pairs
each of which consists of a creation operator (a¯−σ(k, t) or c¯σ′(t)) and a destruction one
(a−σ(l, t) or cσ(t)) for the electrons of one and the same type. The mean-field approxima-
tion can be applied to the T -product of these four operators in the following manner〈
TC [a¯−σ(l; t)a−σ(k; t)cσ(t)c¯σ′(t′)]
〉 ≈ 〈a¯−σ(l; t)a−σ(k; t)〉 〈TC [cσ(t)c¯σ′(t′)]〉
with
〈a¯−σ(l; t)a−σ(k; t)〉 = δkl 〈a¯−σ(k)a−σ(k)〉 = δklna(k),〈
TC [cσ(t)c¯σ′(t′)]
〉
= iGcc¯σσ′(t− t′).
As the result we have
Gaca¯c¯σσ′ (k, l; t− t′) ≈ −δkl[1− na(k)]Gcc¯σσ′(t − t′), (30)
8 Nguyen Van Hieu, Nguyen Bich Ha, and Nguyen Van Hop
where na(k) is the density of the electron with the momentum k and spin projection +σ
or −σ in the lead “a” at the given temperature
na(k) =
e−βEa(k)
1 + e−βEa(k)
,
and similarly for Gbcb¯c¯σσ′ (k, l; t− t′). Applying the mean-field approximation to each of the
others among above-mentioned multi-electron Green functions in any manner we always
obtain the vanishing mean values in the lowest order the perturbation theory with respect
to the effective tunneling coupling constants Va,b(k). Note that these functions enter the
r.h.s. of the equation (29) with the coefficients of the second order with respect to the
effective tunneling coupling constants. This means that in this second order they do not
give contributions. Thus in the second order approximation the equation (29) is simplified
and becomes[
i
d
dt
− (E + U)
]
Hcc¯σσ′(t− t′) =nδσσ′ δC(t − t′) + Γ(t − t′)
+
∫
C
dt′′Σ(2)(t− t′′)Hcc¯σσ′(t′′ − t)
−
∫
C
dt′′Σ(3)(t− t′′)Gcc¯σσ′(t′′ − t),
(31)
where
Γ(t − t′) =
∑
k
[
V ∗a (k)
〈{a−σ(k)cσc+−σ , c+σ′}〉SEa(k)(t− t′)
−Va(k)
〈{c−σcσa+−σ(k), c+σ′}〉S2E+U−Ea(k)(t − t′) + (a→ b)] , (32)
Σ(2)(t− t′) =
∑
k
{
|Va(k)|2 [2SEa(k)(t− t′) + S2E+U−Ea(k)(t− t′)] + (a→ b)
}
, (33)
Σ(3)(t− t′) =
∑
k
{
na(k) |Va(k)|2 [SEa(k)(t− t′) + S2E+U−Ea(k)(t− t′)] + (a→ b)
}
. (34)
Note that in the r. h. s. of the formulae (32)-(34) there appear the crossing terms
containing S2E+U−Ea(k)(t− t′). They must disappear in the noncrossing approximation.
To proceed further we note that〈{a−σ(k)cσc+σ , c+σ′}〉 = −δσσ′ 〈a−σ(k)c+−σ〉 ,〈{c−σcσa+σ (k), c+σ′}〉 = −δσσ′ 〈a−σ(k)c+−σ〉∗ , (35)
where
〈
a−σ(k)c+−σ
〉
is a limiting value of the Green function Gac−σ−σ(k; t):〈
a−σ(k)c+−σ
〉
= iGac−σ−σ(k; +0). (36)
For evaluating the vertex (32) in the second order with respect to the tunneling coupling
constants Va,b(k) we calculate the limiting value (36) in the first order. Introduce the
Dyson Equations for Green Functions of Electrons in Open Single-level Quantum Dot 9
Fourier transformations of the Green functions, for example
Gac¯(k; t) = 12pi
+∞∫
−∞
dωe−iωtG˜ac¯(k;ω),
SEa(k)(t) = 12pi
+∞∫
−∞
dωe−iωtS˜Ea(k)(ω),
Gcc¯(t) = 12pi
+∞∫
−∞
dωe−iωtG˜cc¯(ω).
(37)
From the equation (18) it follows that
G˜ac¯(k;ω) = Va(k)S˜Ea(k)(ω)G˜cc¯(ω). (38)
For deriving G˜ac¯(k;ω) in the first order with respect to the constant Va(k) it is enough to
use the expression of G˜cc¯(ω) in the case of the vanishing tunneling coupling constant and
have
Gac¯(k;ω) =Va(k)
[
na(k)
ω − i0− Ea(k) +
1− na(k)
ω + i0−Ea(k)
]
+
1
Z
[
e−βE
ω − i0−E +
e−β(2E+U)
ω − i0−E − U +
1
ω + i0−E +
e−βE
ω + i0−E − U
]
,
(39)
Z = 1 + 2e−βE + e−β(2E+U)
It is easy to calculate the limit
Gac¯(k; +0) = lim
ε→+0
1
2pi
+∞∫
−∞
dωe−iωεG˜ac¯(k, ω). (40)
by using the residue theorem and obtain〈
a−σ(k)c+−σ
〉
= −νa(k)Va(k), (41)
where
νa(k) =
1
Z
{
e−βE − [1 + e−βE ]na(k)
E − Ea(k) + e
−βE e
−β(E+U) − [1 + e−β(E+U)]na(k)
E + U −Ea(k)
}
, (42)
Therefore the formula (32) can be rewritten in the following manner
Γ(t− t′) =
∑
k
{
|Va(k)|2 νa(k)[SEa(k)(t− t′)− S2E+U−Ea(k)(t− t′)] + (a→ b)
}
. (43)
In many previous works this vertex was omitted.
10 Nguyen Van Hieu, Nguyen Bich Ha, and Nguyen Van Hop
5. CONCLUSION AND DISCUSSION
In the second order approximation with respect to the effective tunneling coupling
constants and the mean-field approximation for the products of 4 operators in the Green
function Gaca¯c¯σσ′ (k, l; t− t′) the system of the two Dyson equations for the complex-time
non-equilibrium Green functions were derived:[
i
d
dt
− E
]
Gcc¯(t− t′) = δC(t − t′) + UHcc¯(t − t′) +
∫
C
dt′′Σ(1)(t− t′′)Gcc¯(t′′ − t′), (44)
[
i
d
dt
− (E + U)
]
Hcc¯(t − t′) =nδC(t− t′) + Γ(t− t′) +
∫
C
dt′′Σ(2)(t − t′′)Hcc¯(t′′ − t′)
−
∫
C
dt′′Σ(3)(t− t′′)Gcc¯(t′′ − t′), (45)
where the self-energy parts Σ(i)(t− t′), i = 1, 2, 3 and the vertex Γ(t − t′) are determined
by the formulae (22), (33), (34) and (43). If t and t′ are the imaginary times t = −iτ ,
t′ = −iτ ′ then this system of equations becomes that of two Dyson equations for the
imaginary time Green functions
Gcc¯(τ − τ ′) = 〈Tτ [cσ(τ)c¯σ′(τ ′)]〉 , (46)
Hcc¯(τ − τ ′) = 〈Tτ [n−σ(τ)cσ(τ)c¯σ′(τ ′)]〉 . (47)
From the equations (44) and (45) and formulae (22), (33), (34) and (43) with the complex
times it is straightforward to derive the Dyson equations for the imaginary time Green
functions(
d
dτ
−E
)
Gcc¯(τ−τ ′) = δC(τ−τ ′)+UHcc¯(τ−τ ′)+
∫
C
dτ ′′Σ(1)(τ − τ ′′)Gcc¯(τ ′′ − τ ′) (48)
(
d
dτ
+E + U
)
Hcc¯(τ − τ ′) =nδC(τ − τ ′) + Γ(τ − τ ′) +
∫
C
dτ ′′Σ(2)(τ − τ ′′)Hcc¯(τ ′′ − τ ′)
−
∫
C
dτ ′′Σ(3)(τ − τ ′′)Gcc¯(τ ′′ − τ ′), (49)
with following self-energy parts Σ(i)(τ − τ ′) and vertex Γ(τ − τ ′)
Σ(1)(τ − τ ′) =
∑
k
{
|Va(k)|2 SEa(k)(τ − τ ′) + |Vb(k)|2 SEb(k)(τ − τ ′)
}
, (50)
Σ(2)(τ − τ ′) =
∑
k
{
|Va( k)|2
[
2SEa( k)(τ − τ ′) + S2E+U−Ea( k)(τ − τ ′)
]
+ (a→ b)
}
(51)
Σ(3)(τ − τ ′) =
∑
k
{
na(k) |Va(k)|2 [SEa(k)(τ − τ ′) + S2E+U−Ea(k)(τ − τ ′)] + (a→ b)
}
(52)
Dyson Equations for Green Functions of Electrons in Open Single-level Quantum Dot 11
Γ(τ − τ ′) =
∑
k
{
|Va( k)|2 νa( k)[SEa( k)(τ − τ ′)− S2E+U−Ea( k)(τ − τ ′)] + (a→ b)
}
(53)
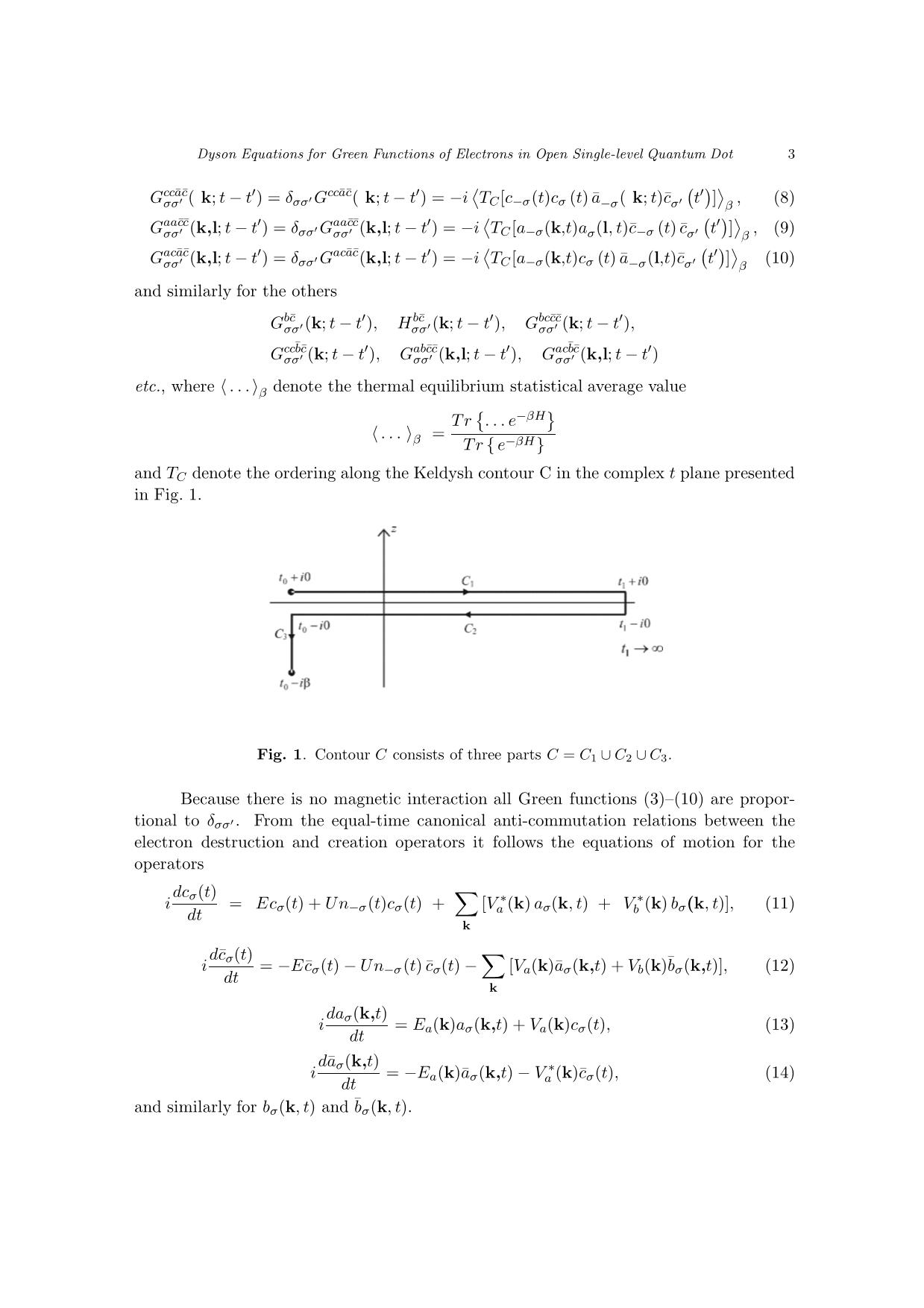
For the study of the real-time Green functions one often considers the case t0 → −∞.
In this limit the contribution of the imaginary-time interval [t0, t0 − iβ] vanishes and the
integral over the contour C becomes the sum of two integral on two straight lines : C1 from
−∞ to +∞ in the upper half plane and C2 from +∞ to −∞ in the lower half plane (Fig.
1). Because each complex time t or t′ can belong either to C1 or C2, each complex-time
Green functions is a set of four functions of the real variable t− t′, for example
G
(
t − t′) =

G(t− t′)11 if t, t′ ∈ C1,
G(t− t′)12 if t ∈ C1, t′ ∈ C2,
G(t− t′)21 if t ∈ C2, t′ ∈ C1,
G(t− t′)22 if t, t′ ∈ C2.
(54)
Therefore the Fourier transform of each complex-time Green function is a set of four
Fourier transforms of four functions of the real time t − t′ and can be represented as a
2× 2 matrix
Gˆ(ω) =
(
G˜(ω)11G˜(ω)12
G˜(ω)21G˜(ω)22
)
,
Hˆ(ω) =
(
H˜(ω)11H˜(ω)12
H˜(ω)21H˜(ω)22
)
.
(55)
From the differential – integral equations (44) and (45) it follows two matrix equa-
tions for the matrices (55):
(ω − E)Gˆ(ω) = 1 + UHˆ(ω) + Σˆ(1)(ω)ηˆGˆ(ω), (56)
(ω −E − U)Hˆ(ω) = n+ Γˆ + Σˆ(2)ηˆ Hˆ(ω)− Σˆ(3)(ω)ηˆGˆ(ω) (57)
where ηˆ is the metric matrix
ηˆ =
(
1 0
0 −1
)
(58)
and Σˆ(1)(ω), Σˆ(2)(ω), Σˆ(3)(ω) and Γˆ(ω) are the matrices of the Fourier transforms of the
self-energy parts and the vertex.
By solving the system of equations (56) and (57) we can derive the analytical ex-
pressions of the Fourier transforms of the Green functions. They will be used for the study
of the physical characters of the QD in subsequent works.
Acknowledgment. The authors would like to express their gratitude to the Ministry of
Science and Technology for support of the National Research Program in Natural Sciences.
REFERENCES
1. Y. Meir, N. S. Wingreen and P. A. Lee, Phys. Rev. Lett. 66 (1991) 3048.
2. S. Hershfield, J. H. Davies and J. W. Wilkins, Phys. Rev. Lett. 67 (1991) 3720.
3. Y. Meir, N. S. Wingreen and P. A. Lee, Phys. Rev. Lett. 70 (1993) 2061.
4. T. K. Ng, Phys. Rev. Lett. 70 (1993) 3635.
5. A. L. Yeyati, A. Martin-Rodero and F. Flores, Phys. Rev. Lett. 71 (1993) 2991.
12 Nguyen Van Hieu, Nguyen Bich Ha, and Nguyen Van Hop
6. T. Inoshita, A. Shimizu, Y. Kuramoto and H. Skaki, Phys. Rev. B 48 (1993) 14725.
7. N. S. Wingreen, Y. Meir, Phys. Rev. B 49 (1994) 11040.
8. L. Craco and K. Kang, Phys. Rev. B 59 (1999) 12244.
9. T. Fujii and K. Ueda, Phys. Rev. B 68 (2003) 155310-1.
10. M.-S. Choi, D. Sa’nchez and R. Lo’pez, Phys. Rev. Lett. 92 (2004) 056601.
11. O. Takagi and T. Saso, J. Phys. Soc. Jpn. 68 (1999) 1997; ibid. 68 (1999) 2894.
12. W. Izumida, O. Sakai and Y. Shimizu, J. Phys. Soc. Jpn. 66 (1997) 717; ibid. 67
(1998) 2444.
13. O. Sakai, S. Suzuki, W. Izumida and A. Oguri, J. Phys. Soc. Jpn. 68 (1999) 1640 .
14. W. Izumida, O. Sakai and S. Suzuki, J. Phys. Soc. Jpn. 70 (2001) 1045.
15. M. E. Torio, K. Hallberg, A. H. Ceccatto and C. R. Proetto, Phys. Rev. B 65,085302
(2002).
16. R. Swirkowicz, J. Barnas and M. Wilczyuski, Phys. Rev. B 68 (2003) 195318.
17. Nguyen Van Hieu and Nguyen Bich Ha, Adv. Nat. Sci. 6 (2005) 1 .
18. J. Konig and Y. Gefen, Phys. Rev. B 71 (2005) 201308(R).
19. M. Suidel, A. Silva, Y. Oreg and J. von Delft, Phys. Rev. B 72 (2005) 125318.
20. L. V. Keldysh, Jh. Eksp. Teor. Fiz, 20 (1965) 1018.
21. K.–C. Chou, Z.–B. Su, B.–L. Hao and L. Yu, Physics Reports 118 (1985) 1.
Received 18 January, 2006.